Category The Enigma of. the Aerofoil

Lanchester offered no quantitative expression for the dependence of lift on circulation. He presented many of his main results in a verbal or pictorial form. A modern textbook of aerodynamics would give a mathematical for­mula to express the central ideas of Lanchester’s theory of lift. The formula would be

Lift = рГУ,

where p is the density of the air, Г is circulation (that is, the value of the integral described earlier), and V is the speed of the wing relative to the un­disturbed air.

It is a matter of some historical interest that this important formula had been published in 1877 by Lord Rayleigh. Rayleigh did not, however, offer it as a law that might govern the lift on an aircraft wing. He reserved that role for the very different formula he had published in the previous year describ­ing the pressure on a flat plate created by a discontinuous flow. How and why did Rayleigh arrive at the рГУ formula? I want to look into this matter and then come back to Lanchester.

The short paper in which the formula appeared was titled “On the Ir­regular Flight of a Tennis Ball.”15 In it Rayleigh posed the question of why a spinning ball sometimes veers to one side in flight: “It is well known to tennis players that a rapidly rotating ball in moving through the air will often deviate considerably from the vertical plane. There is no difficulty in so projecting a ball against a vertical wall that after rebounding obliquely it shall come back in the air and strike the same wall again” (344).

In posing his question Rayleigh would have been thinking of the old game of royal or real tennis, which was played indoors and involved a net strung between two walls. In this form of tennis the players are permitted to use the walls and hence produce the effect that Rayleigh mentioned.16 Real tennis courts only existed in a few country houses and ancient universities, but there were (and still are) courts in Cambridge. It was Rayleigh’s chief recreation as an undergraduate.17 Rayleigh rejected the idea that the trajectory of the ball deviated from a straight line because the ball “rolled” on a cushion of con­densed air that had formed in front of it. That, he noted, would produce a deflection in the wrong direction. The correct answer, he said, had been given in a qualitative form by the Berlin scientist Gustav Magnus in 1852. Magnus had been interested in artillery shells rather than the more gentlemanly tennis ball.18 The present paper, said Rayleigh, was designed to supplement Magnus’ answer with a mathematical formulation.

To simplify the problem Rayleigh assumed that the ball was stationary and the air flowed uniformly past it. If the ball is not spinning, all the forces, frictional and otherwise, will be in the direction of the air stream. To produce a deviation in the trajectory there must be a lateral force. This force depends on the rotation of the ball, which, by friction between the ball and the air, produces what Rayleigh called “a sort of whirlpool of rotating air” (344). If the whirlpool is combined with the uniform stream, the velocities oppose one another on one side of the ball and augment one another on the other side. This velocity difference produces a pressure difference and hence a side force. This time the force is in the correct direction. “The only weak place in the argument,” said Rayleigh, “is in the last step, in which it is assumed that the pressure is greatest on the side where the velocity is least. The law that a diminished pressure accompanies an increased velocity is only generally true on the assumption that the fluid is frictionless and unacted upon by external forces; whereas, in the present case, friction is the immediate cause of the whirlpool motion” (345). The law relating diminished pressure with increased velocity was Bernoulli’s law, and Rayleigh was rightly sensitive to
the preconditions of its legitimate use. Despite the questionable character of this step in the argument Rayleigh continued to develop the analysis in terms of a circular cylinder “round which a perfect fluid circulates without molecu­lar rotation.” What was meant by “molecular” here? Rayleigh was explicitly working with an ideal or perfect fluid, so the “molecules” would actually be fluid elements. Rayleigh would not have been confusing them with the mol­ecules of the chemist. He was simply postulating an irrotational flow of an incompressible, inviscid fluid with circulation.

Rayleigh proceeded to write down the stream function for the flow he was postulating. He was able to do this by adding together the stream functions for the two components of the flow, namely, the stream functions for a uni­form flow round a cylinder and for a vortex round a point. The formula he used was, in his notation,

r sine + ySlogr,

where r and 0 are the polar coordinates of any point in the fluid and a is the radius of the cylinder. The two symbols a and в are constants proportional to the velocity of the free stream and the circulation, respectively. Examina­tion of Rayleigh’s formula shows that it can be related to the examples I have discussed in previous sections. The first part is the stream function that was discussed in chapter 2 for the flow around a circular cylinder. The second part, involving the log term, was introduced earlier in this chapter as the stream function of a vortex.

Rayleigh used this formula to arrive at an expression for the velocity and hence the pressure at any point on the surface of the cylinder. The lateral component was integrated around the circular cylinder to give the resultant lateral force. The expression that Rayleigh arrived at was

2n

I (P – P0 )a sinede = -2mxp,

0

where p is the pressure at the surface of the cylinder and p0 is the pressure at infinity. The other symbols have the meanings already assigned. The conclu­sion indicated by the final term of this equation was that the lateral force causing the irregular flight of the tennis ball was “proportional both to the velocity of the motion of circulation, and also to the velocity with which the cylinder moves” (346). This is essentially the result on which Lanchester’s analysis depended, and the right-hand side of the above equation is equiva­
lent to the law relating lift to density, circulation, and velocity. The minus sign on the right-hand side of the above equation indicates direction, the circulation is Г = 2пв, while the free-stream velocity is V = a. Rayleigh took the density of the air to be of unit value, which explains why the term p did not appear in his final formula.

Having arrived at the lateral force on the tennis ball, Rayleigh asserted without argument (but citing the authority of Kelvin) that, under the sim­plified conditions of the example, the trajectory of the tennis ball would be along the arc of a circle, the motion having the same direction as the circula­tion.19 Rayleigh’s analysis was taken up and extended by Greenhill in a paper published in 1880 in which the equation of the trajectory was deduced in an explicit way.20 Further, whereas Rayleigh had ignored external forces such as gravity, Greenhill reintroduced this complication and showed that its effect was to give the trajectory the form not of a circle but of a trochoid.21

How seriously did Rayleigh take his analysis of the tennis ball and, by implication, the artillery shell? His aim in the paper, he explained, had been to “solve a problem which has sufficient relation to practice to be of interest, while its mathematical conditions are simple enough to allow of an exact so­lution” (345). These words reveal the element of judgment that entered into the exercise. Relevance was being traded with simplicity. A balance was being struck, but Rayleigh evinced some doubts about the terms of the exchange. His final words in the paper referred back to what he had called the weak links in the argument. The behavior of a real fluid would give rise to a wake behind the ball, and this aspect of the flow finds no recognition in the analysis. Ray­leigh thus ended his paper with a warning: “It must not be forgotten that the motion of an actual fluid would differ materially from that supposed in the preceding calculation in consequence of the unwillingness of stream-lines to close in at the stern of an obstacle, but this circumstance would have more bearing on the force in the direction of motion than on the lateral compo­nent” (346). The informed reader of Rayleigh’s paper would know that there was a mathematical approach that could represent this “unwillingness” of the streamlines to close in behind an obstacle. Such an analysis was provided by the theory of discontinuous flow and free streamlines. Perhaps this would do more justice to the complex reality of the case. Explicitly, then, the reader was given a circulation analysis but, implicitly, was being directed toward the theory of discontinuous flows.22

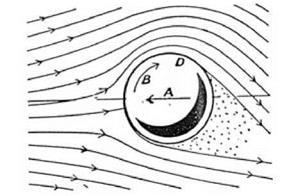
Lanchester also had an account of the swerving tennis ball in his book on aerodynamics. His account did not invoke the circulation theory but ap­pealed to the discontinuity theory, that is, to the picture of Kirchhoff-Rayleigh

flow. Lanchester’s discussion of the spinning ball was given in a section of his book titled “Examples Illustrating Effects of Discontinuous Motion.” The diagram he used to illustrate his analysis is given here in figure 4.9. The figure shows a ball moving through a viscous fluid, such as air, where the direction of motion is shown by the arrow A, and the rotation by arrow B. In this case the ball will veer upward. Like Rayleigh, Lanchester drew attention to the in­crease in the speed of the air on one side (here the top) and its diminution on the other side (the bottom). Where Rayleigh merely hinted, Lanchester was explicit that the flow round the ball creates dead fluid behind it. He argued for this concept on experimental grounds. The effect of the spin was then said to modify the shape of the dead air, as is shown in the figure. Lanchester claimed that at the top of the dead-air region, the spin helps to expel the dead air. At the bottom, the spin helps to draw in the dead air and spread it along the lower surface of the ball. This action was said to have the effect of giving the ball an upward thrust.

In retrospect, familiarity with the circulation theory of lift makes it easy to assimilate the case of the spinning tennis ball and the case of the wing and to see the possibilities inherent in treating both of them as involving circulation. It is evident that for Rayleigh and Lanchester the situation was not clear cut and both inclined toward an eclectic account. In broad terms it can be said that Rayleigh used the discontinuity theory to explain wings but the circula­tion theory to explain tennis balls. Lanchester did the opposite and used dis­continuity theory to explain the swerve of tennis balls and circulation theory to explain the lift of wings.

Professor or Practical Man?

Not long after its publication, the first volume of Lanchester’s Treatise be­came the topic of a seminar discussion in Gottingen, and Lanchester was approached for permission to produce a German translation. He received a letter to that effect from Prof. Carl David Runge. At first Lanchester had no idea who Runge was, but inquiries soon revealed that he was an emi­nent applied mathematician. He had done work on methods of numerical approximation used to solve differential equations as well as having discov­ered empirical formulas to predict spectral lines.23 Runge had been brought from the technische Hochschule in Hanover to the university in Gottingen at the behest of Felix Klein. During the session of 1907-8 Runge had joined Prandtl in giving a seminar on hydro – and aeromechanics. They discussed Lanchester’s book, and Runge penned his letter to Lanchester in March 1908. In September, Lanchester visited Runge in Gottingen to discuss the transla­tion, and they became lifelong friends. Runge was impressed by Lanchester’s vigor and humor, which were very different from the demeanor of the aca­demic Englishmen he had met before. Lanchester was a guest of Runge and his wife and amused their young sons by flying model gliders. Lanchester recalled that one evening Prandtl and von Parseval (the airship designer)24 “blew in” for a beer. A convivial time was had, though communication was limited by Lanchester’s lack of German and Prandtl’s lack of English. Runge, by contrast, spoke English with a perfection that amazed Lanchester.25 The German translation of the Treatise was a collaborative effort by Runge and his wife. The first volume was published in 1909.26

Lanchester’s appointment to the Advisory Committee for Aeronautics in 1909 is not difficult to understand in general terms—though exactly how it came about remains unknown. He had published a substantial work on aero­nautics and was an engineer with a proven record of innovation. His knowl­edge of engine design was considerable, and the difficulty of producing light, powerful, and reliable engines remained a major stumbling block to progress in aviation. Lanchester grumbled retrospectively that Glazebrook thought that this was his only area of expertise: “he always treated me as if I was ap­pointed purely for my knowledge of the internal combustion engine.” In the hope of being taken seriously on a broader front, Lanchester had distributed six copies of his treatise Aerial Flight to his fellow committee members. Ray­leigh responded with an appreciative letter and gave Lanchester four volumes of his collected papers in return. Of the rest, said Lanchester, only one ac­knowledged the gift, while the others “ignored it entirely.”27 Despite these slights Lanchester worked hard, both for the main committee and for the two subcommittees on which he served: the Engine Sub-Committee and the Aerodynamics Sub-Committee. His biographer described how Lanchester would attend some forty to fifty meetings a year as well as taking on the re­sponsibility of writing a significant number of papers published as Reports and Memoranda.28

While Lanchester’s appointment to the ACA is intelligible, his position on the committee was anomalous. He was a representative of the world of practical engineering on an administrative and scientific body dominated by influential Cambridge academics. He was not the only engineer or the only nonacademic or, indeed, the only non-Cambridge man on the com­mittee, but (the military representatives aside) the others were more closely integrated into academic life. Joseph Petavel was a professor of engineering at Manchester and Horace Darwin, of the Cambridge Scientific Instrument Company, had close connections with the university. This left O’Gorman and Lanchester as the only authentic examples of what the British called “practi­cal men,” but this did not spare them from the hostility that was directed against the “theoreticians” of the Advisory Committee.

Lanchester’s treatise was published before his appointment to the hated committee, so how was it received in the aeronautical and engineering press? The first volume was reviewed in Aeronautics in February 1908. The review was unsigned but was probably written by the editor, J. H. Ledeboer. The reviewer recommended Lanchester’s book for thorough study by all those who take an interest in aeronautical problems, “since it includes a mass of valuable theory.” The aircraft designer could gain “many a hint” from the book. The reviewer did not, however, identify the cyclic theory of lift among the “mass.”29 The anonymous reviewer in the Aeronautical Journal likewise greeted Lanchester’s first volume as a valuable, but difficult, contribution to aerodynamical theory and also failed to convey to the reader the cyclic char­acter of the flow postulated in Lanchester’s book.30

The same pattern was repeated in the anonymous reviews published in the Engineer, Engineering, and the Times engineering supplement.31 Florid congratulations were offered by the reviewer, but there was a signal failure to convey the actual content of the theory of lift. The words “circulation” or “cy­clic” did not feature in any of the discussions. There were also warning signs suggesting that the reviewers might have opposed the theory had they grasped it. Thus, the reviewer in the Times spoke of “doubtful hydrodynamic analo­gies” (6), while the reviewer in the Engineer noted that “Evidently the author has a deep admiration for the work of Horace Lamb” (617) and went on to say that Lanchester “somewhat naively” described how he had added the hydro­dynamic interpretation to his original ideas in order to give it a more secure basis. The naivety, presumably, lay in Lanchester’s failure to realize that, in certain quarters, invoking Lamb would reduce rather than increase credibil­ity. The reviewer in Engineering also took issue with Lanchester’s diagrams: “The diagram given on page 145 seems to indicate that particles originally in contact with the lower surface of the plane finally reach the upper by describ­ing circular paths round the edge. The true condition of affairs is, we believe, much more nearly represented by a diagram given later in the volume—viz. on page 266” (461). The complaint is revealing. As Lanchester had explained on the page before the diagram favored by the reviewer, at that point in the discussion “the flow is of the Rayleigh-Kirchhoff type” (265). The reviewer was responding to, and endorsing, a diagram that shows a discontinuous flow—precisely the picture that Lanchester was challenging.

Most of the “practical men” who read Lanchester initially extracted from him not the idea of circulation but the neo-Newtonian idea of “sweep.” The realization that circulation played a central role in Lanchester’s thinking came later. For example, Arthur W. Judge in his eclectic Properties of Aerofoils and Aerodynamic Bodies followed Lanchester in giving an account of both circula­tion and sweep, including a reproduction of some of the diagrams showing trailing vortices.32 Judge’s book was published in 1917, rather than being an immediate reaction, so there was more time for Lanchester to have dissemi­nated his ideas.33 Nevertheless, Judge did little to integrate the circulatory theory with empirical data, despite his references to some of the Gottingen publications.34

The second volume of Lanchester’s treatise was reviewed in Aeronautics a year after the first volume, and this time the hand of the editor was sig­naled by the initials J. H. L.35 He expressed “whole-hearted admiration” for the work but soon took the discussion into the vexed domain of the relation between theory and practice. “The present work once again raises the oft – debated question of the advantages in aeronautical research of the theoretical or mathematical and practical methods” (6). In the reviewer’s opinion, “vic­tory” rested with the practical methods. Admittedly, he went on, people such as Bryan, Rayleigh, “and, we may now add, Lanchester” show the path along which research is to proceed, but the fact remained that “the most success­ful machine of all,” that of the Wright brothers, was built without the aid of mathematical formulas. This, then, was, “the main reproach we could make against Mr. Lanchester’s work: it is far too theoretical” (6).

The designer J. D. North had apparently arrived at a similar conclusion. He later recalled that he had not found Lanchester’s book as useful as the more empirical, data-rich volumes of Eiffel.36 This opinion was not universal, but clearly many people saw Lanchester in this way, that is, as a representative of an overly theoretical approach. In February 1914, Herbert Chatley wrote an article in Aeronautics designed to head off the reading of Lanchester’s book as just another example of mathematical “x-chasing.”37 Chatley acknowledged that there was controversy in the technical press about the value of applying mathematics to aeronautical problems. One dimension of the problem, he said, was generational. Older engineers had been trained practically and re­sented the attempts of younger colleagues “to dominate engineering practice with mathematical theorems.” Chatley wanted to do justice to both sides and to get the competing parties to see that the problem did not have a black or white answer. The issue, he said, was always one of degree.

Chatley illustrated his conclusion by an example: “There are three books published in England by men with big reputations dealing with the theory of aviation, viz. Lanchester’s ‘Aerial Flight,’ Bryan’s ‘Stability in Aviation,’ and Greenhill’s ‘Dynamics of Mechanical Flight.’ To those unfamiliar with math­ematics these three books are classed together and, I imagine, are regarded by the majority of practical aeronautical engineers as beyond the limits of practi­cal application” (46). It was a mistake, he said, to treat these books as three of a kind. Lanchester’s book was not obscure and was of the greatest use to designers; Bryan’s book was, indeed, mathematically much more demanding, but it was genuinely engaged with real problems; Greenhill’s book, however, was quite different. It was even more mathematically difficult than Bryan’s but “very little of it has at present any application to practical problems.” As well as making discriminations of this kind, said Chatley, both sides, the prac­tical men and the mathematicians, must recognize the weakness in their own positions. The former must give up reliance on “mechanical instinct,” while the latter must “assimilate more complex conditions” rather than “elaborate hypotheses on too simple foundations” (46).

Chatley was seeking a compromise, but this desire was wholly foreign to C. G. Grey, editor of the Aeroplane. On September 12, 1917, Grey described Lanchester as “several government officials rolled into one, and a mathemati­cian, and an advisory expert, and several other equally exalted things at the same time.”38 Grey took pleasure in baiting Lanchester, who held no academic post, by calling him “Professor.”39 While granting that Lanchester’s books had their “lucid moments,” Grey claimed that, overall, they were “utterly ununderstandable. . . to the ordinary man, and even to some trained math­ematicians.” He called on Lanchester to mend his ways: “No! Mr. Lanchester. Forget your professorship for a while. Be a practical engineer” (158). In an article headed “On a Professor,” Grey described Lanchester’s main publica­tions in unflattering terms: “He has written two ponderous tomes, entitled ‘Aerodynamics’ and ‘Aerodonetics’ respectively, which profess to be math­ematical expositions of the theory of flying. On these volumes his reputation in aeronautics was founded. Not being a mathematician myself I can only ac­cept the statement of others better informed, who say that these expositions are not mathematics in the accepted sense of the term, but some science of symbols invented by Professor Lanchester.”40

What had called forth this invective? The answer is that Lanchester had ventured into what an editorial in Flight had called “the cesspool of politics.”41 He had been goaded into responding to articles that Grey had published in the Sunday Times and the Observer on July 29, 1917. Lanchester’s reply was called “A Campaign of Slander.” It appeared on August 15, 1917, in the short­lived journal Flying.42 He said Grey was indulging in “a venomous and un­restrained attack on the Air Board and upon the two branches of the Air Service.”43 These attacks repeated earlier accusations made by Pemberton Billing and Joynson-Hicks in Parliament—accusations, said Lanchester, that had been thoroughly investigated and found to be groundless. Lanchester was referring to the “murder” charge leveled at the government and to the Bailhache inquiry whose final report had been published on November 17, 1916. “No documents,” said Lanchester, “could constitute a worse indictment of the self-appointed critics, or a greater vindication of the ability, character and honour of those attacked, unless possibly that document be the record of the proceedings themselves. . . . It is probable that in our time no public enquiry has been called upon to investigate charges so recklessly made and based on so slender a foundation” (51).

Now, a year later, Grey was repeating the old complaints. Notice, said Lanchester, how those making the attacks were always “wily enough to avoid putting them in a form which would permit of their being challenged in the Courts” (51). They always expressed themselves in a vague and general way. They talked of “appalling waste,” “official designers,” a “little clique,” etc. It was a case, said Lanchester, of simply throwing mud in the hope that some­thing would stick. The ground of the attack, as before, was the allegation of official incompetence and favoritism. Even the old BE type, an aircraft cur­rently being replaced, was still under attack. “Its present survival in active service,” said Lanchester, “is talked about as an offence attributable to some interested and evil-disposed persons in authority. The whole tenor of these long abusive articles is based upon the existence of machines, any machines whatever, of official design, when (it is alleged) far better machines of propri­etary design are available” (51).

This was the nerve of the issue: the existence of official designs. The ques­tion with which we are faced, said Lanchester, “is why and to whom is the said design an offence?” (52). Had these machines failed to perform their re­quired duties? Were there any better designs available when the BE2C was put into manufacture? The answer to both questions, said Lanchester, “is clearly in the negative” (52). The BE type had been the backbone of the reconnais­sance service for three years of war. It had to bear the blame for its shortcom­ings as a fighter and for being not quite the equal in speed and climb to the Fokker, but, insisted Lanchester, it had acquitted itself “in a manner which is little short of astonishing” (52).

Lanchester then addressed Grey’s complaints about engine shortages and the mobilization of manufacturing capacity in order to improve aircraft sup­ply. In Lanchester’s opinion, none of Grey’s dramatic remedies could stand up to scrutiny. Grey had no understanding of real conditions, and his ideas about economics were naive. Grey was responding to an inevitable feature of large-scale production but was treating it as if it were a defect that could be swept away. Thus, said Lanchester,

He condemns offhand everything which is not the best. If a new machine is produced to-day which is better in its performance. . . on trial than some­thing in service, then the latter is at once condemned, and the people who tolerate its use are obvious fools or incompetent rogues! It is spoken of as murder still to employ the B. E.2c for reconnaissance, or an 80-h. p. scout as a fighter. Mr. Grey has evidently had no experience of quantity production, otherwise he would know that it is always the case, so long as there is progress, that the latest and the best designs will not be available in sufficient quantity. It cannot in the nature of things be otherwise. (53)

Grey may have been the mouthpiece of the aircraft manufacturers, but in Lanchester’s opinion he betrayed no understanding of the system of manu­facture and development.

Lanchester returned to these themes later in 1917, in the December issue of Flying. The title of Lanchester’s article was “The Foundation Stones,”44 and he set out to explain the scientific and research basis on which airplane de­sign depended. The airplane as we know it today, argued Lanchester, doesn’t merely depend on the experience of the draughtsman, the intuition of the designer, or the cunning of the craftsman but “is the ultimate outcome of scientific research” (354). Theory, both mathematical and otherwise, played a role, while engineering and physical research, carried out in the workshop, the laboratory, and with full-scale aircraft, consolidated the results already explored theoretically. Lanchester’s aim, however, was not simply to draw attention to the diversity of the scientific foundations of aeronautics. The different contributions had to be properly coordinated: “In order to secure continued progress it is vitally necessary that forces of many different kinds should act in concert, and nothing but evil can result from the worker in any particular field trying to encroach on other fields” (354). Lanchester listed the various contributors and delimited the proper scope of their activities. The mathematician may be useful but is “utterly incompetent” when it comes to “specifying or designing an aeroplane” (345). The mathematician’s field is im­portant but narrow. Unfortunately, many mathematicians are men of “child­like simplicity” and fail to understand that there is anything beyond their symbols. Physicists also have a vital role but “only one degree less narrow” than that of the mathematician. “For the physicist to invade territory outside his own ken would certainly not lead to satisfactory results” (354). The engi­neer is responsible for design and construction. “His job is frequently that of a buffer.” He has to listen to both the mathematician and the physicist but he must also be responsive to the requirements of the user, and pay attention to economic considerations and, in times of war, to shortages of materials and the problems of substituting one material for another. The pilot also had a legitimate claim to be heard, as did the military and naval authorities who ordered the aircraft and were responsible for its deployment.

Lanchester had no illusions about a system of this kind. As a realist he knew there would always be clashes of interest. They were inevitable and could not be wished away, but they were not always bad in their effect. In a tilt at the press campaigns he declared: “Now there are many who think that fric­tion in the management of our Air Services as between one department and another, or between different departments and manufacturers, or between the military authorities at the Front and those at home, etc., is essentially a sign of bad management and muddle. No such idea is justified” (354).

Theoretically, if a single “autocrat” were in control, friction might be avoided, but even then the autocrat would have to defer to those with spe­cialized knowledge. In reality the answer lay in the proper division of labor and clear, long-term policies. “The greater part of the difficulties that have arisen in the past have been due to two causes, one a failure to define prop­erly the spheres or fields of activity of the different contributory factors; the other an absence of foresight and far-sighted policy, both in detail and in the gross” (354).

As an example of a failure of foresight, Lanchester pointed to the attacks on mathematicians and physicists and their condemnation as impractical. At times these specialists may have made themselves too prominent, but their contributions are indispensable. The best way to achieve the desired har­mony between the different factions “can only come from each man putting up as stout a case as he can for his particular views,” while being prepared to listen to the views of others. Such negotiations have something of the char­acter of a game of chess. We should not forget that when a mistake is made, its consequences “may often be so remote as to defy immediate analysis,” or that the winning move “may have every appearance at the time it is made of being a blunder” (355).

In Lanchester’s opinion, “indiscriminate Press criticism and stump ora­tory” only served to distract from the serious business of the war (355). Jour­nalists who thought they were omniscient, he went on, merely traded on the ignorance of their fellows. The proper way to proceed was shown by the late E. T. Busk, who acted as a “bridge between the scientific man, the engineer and the pilot” (356). In the period leading up to the war, opinion was divided on the value of the inherent stability of an aircraft. Physicists and mathema­ticians, said Lanchester, strongly supported stability, while many pilots had been against it (as had the Wright brothers). The military authorities didn’t know what to do. Busk resolved this dispute and “proved the value of in­herent stability” (356). Lanchester accepted that since Busk’s work, in 1914, knowledge of how to balance the relative virtues of stability and instability had been deepened. Despite these subsequent advances, the “BE2C machine was the immediate outcome of his work, and it is worthy of note that every Zeppelin brought down in night flying in this country was brought down by a machine of this type. For a long time it was the only machine which could safely be sent up or flown in the dark. Beyond this, for the first twelve months of the war, and more (up to the spring of 1916), nearly two thirds of the total enemy aeroplanes brought down on the Flanders front were brought down by the BE2C” (356).

Lanchester was the only member of the Advisory Committee for Aero­nautics to engage in public with the likes of Grey and Pemberton Billing. He was appalled at their activities and had the civic courage to say so. He was also disturbed by the mounting evidence that Billing was conducting his campaign with the help of inside information provided by a member of the Advisory Committee itself. That member, Lanchester concluded, was the naval rep­resentative Murray Seuter. Lanchester complained in letters to A. J. Balfour and G. A. Steel at the Admiralty that Seuter was a slacker who did not pull his weight and abused his position of trust. Lanchester’s suspicions had been aroused when he had shown Seuter an article he intended to publish in one journal but had then decided to publish elsewhere. Within an hour of showing the document to Seuter, one of Billing’s supporters had rung up the original (that is, the wrong) publisher to raise objections. “I think,” said Lanchester pointedly, “Commodore Seuter should be given a change of air.”45

Although some practical men treated Lanchester as if he were a mere theoretician, in his own understanding he was very much the engineer. He had a sharp sense of the proper relationship of the engineer to the mathema­tician and physicist and of the differences in their perspective. I shall now look at how this sense of difference was reciprocated by mathematicians and physicists themselves. I review, in roughly chronological order, a sequence of critical responses to the circulatory theory of lift, as they were advanced by the high-status, mathematically sophisticated British experts in aerodynam­ics, and I identify all of the main objections. Running against the flow of these objections were some experimental results that might have worked in Lanchester’s favor, but, strangely, these had little impact. Why this was so is part of the problem to be addressed.

The influential vision of the turn-of-the-century Cambridge school of math­ematical physics, as Lamb, Love, and others presented it, stood in contrast to the German idea of technical mechanics. This body of work came out of the great system of German technical colleges or technische Hochschulen, such as that at Charlottenburg, or in Munich where Prandtl had studied, or Hanover where both Prandtl and Runge had taught before their call to Gottingen. A representative example of this style of work is provided by August Foppl’s influential lectures on technical mechanics. His multivolume and vastly pop­ular Vorlesungen uber technische Mechanik was published in many editions around the turn of the century. Foppl originally worked in industry and had spent a number of years teaching in a trade school. He later rose to become the professor of theoretical mechanics at the Munich Hochschule and the di­rector of their materials laboratory.47 A versatile mathematician, Foppl had written the first book introducing Maxwell’s work on electromagnetism into Germany. The book was later revised and coauthored with the experimental physicist Max Abraham, and it is known that one student who was influenced by it was the young Einstein.48 Foppl had been Prandtl’s teacher at Munich and had supervised his doctoral research on the buckling of loaded beams.49 In 1909 Prandtl married Foppl’s eldest daughter, Gertrud. In Prandtl’s biog­raphy, written by his own daughter, there is evidence of a certain tension between Prandtl and his father-in-law, occasioned by the older man’s au­thoritarian attitudes, but there was no lack of scientific respect.50 Ludwig Foppl, who, along with Abraham and von Karman, had been in the audience at Cambridge when Lamb spoke, was one of August Foppl’s two sons. The other son, Otto Foppl, worked with Prandtl on wind-tunnel experiments in Gottingen. Some of Otto Foppl’s work is discussed in a later chapter; for the moment, however, the concern is with August Foppl (fig. 5.5).

What did the many readers of Foppl’s published lectures on technische Mechanik learn about the status of their field as they imbibed its carefully graded and expertly presented content? First, they learned that mathematics was a means to an end, rather than an end in itself. In the introduction to the first volume, Foppl wrote that mechanics makes extensive use of mathematics, but as an auxiliary. Mathematical techniques, he said, were simply the clothing in which the body of knowledge was garbed. The point was reiterated at the beginning of the more mathematically demanding third volume, but this time with more stress on just how important, on occasion, these aids could be:

Analytische Entwicklungen betrachte ich immer nur als ein Mittel zur Er – kenntnis des inneren Zusammenhangs der Thatsachen. Wer auf sie verzichten wollte, wurde das scharfste und zuverlassigste Werkzeug zur Verarbeitung der Beobachtungsthatsachen aus der Hand geben. (1900a, viii)

I only consider analytical processes as a means for understanding the intimate interconnections of the facts. Those who want to renounce them are letting go of the sharpest and most dependable tools for working with the facts of observation.

Mathematics provided what Foppl called Hilfsmittel and Werkzeuge, “aids” and “instruments.” Foppl’s language is important here. There had been in-

tense, not to say wearisome, debate in German academic circles over whether mathematics was to be seen as a Hilfswissenschaft or as a Grundwissenschaft with respect to technology.51 Was it an auxiliary to, or a foundation of, tech­nology? The debate was really a coded argument over the status of math­ematicians in the technical college system and their role in the education of engineers. Foppl was signaling that mathematicians had to earn their living by making themselves useful to engineers. The function of mathematics was to further technology and engineering.

The first chapter of volume 1 of the Vorlesungen was devoted to the ori­gin and goals of mechanics. Foppl acknowledged that mechanics was part of physics and, like all the sciences, was grounded in experience. To grasp experience, he argued, it was always necessary to work with simplified, easily imagined “pictures” (Bilder) of reality. The ideas of a point particle and a rigid body were two such pictures. Both were valuable and had their appropriate range of application, but they must not be mistaken for physical realities.52 Foppl also drew a distinction between the Naturforscher and the Techniker— the natural scientist and the engineer. His book was for the latter, not the former, and dealt with a mode of knowledge having special characteristics that differentiated it from natural science in general.

Bei der technischen Mechanik tritt als bestimmender Beweggrund fur ihre Fassung zu der Absicht einer Erforschung der Wirklichkeit. . . noch die an – dere Absicht, ihre Lehren nutzbringend in der Technik zu verwerthen. (11)

In the case of technical mechanics there is a definite motive for its approach over and above the intention to investigate reality. . . and that further inten­tion is that its theories be usefully applicable in technology.

Foppl was just the sort of utilitarian, applied mathematician from whom Lamb and Love had distanced themselves. Indeed, Lamb’s typology might have been expressly contrived to ensure that the Cambridge school did not get caught in the cross-fire between the champions of mathematics as Hilfswis- senschaft and as Grundwissenschaft. Be this as it may, Foppl certainly didn’t have the Cambridge tone. His technical mechanics was not natural philoso­phy. Furthermore, Foppl differentiated technical mechanics from mechan­ics in general because there are many cases when the general doctrines of mechanics do not, or do not yet, provide rigorous answers to the questions that have to be confronted by the engineer. Natural scientists and engineers, he said, stand in a wholly different relationship to these cases: “solchen Fallen steht aber der Naturforscher anders gegenuber als der Techniker” (11). The engineer must produce an answer and must forge concepts to deal with the problem. The natural scientist can wait for inspiration or more information; the engineer cannot:

Der Techniker dagegen steht unter dem Zwange der Nothwendigkeit; er muss ohne Zogern handeln, wenn ihm irgend eine Erscheinung hemmend oder fordernd in den Weg tritt, und er muss sich daher unbedingt auf irgend eine Art, so gut es eben gehen will, eine theoretische Auffassung davon zurechtle – gen. (11-12)

The engineer, by contrast, is subject to the force of necessity. He must, with­out delay, deal with the matter when some phenomenon interferes and inter­poses itself in his path. He must, in some way or other, arrive at a theoretical understanding of it as best he can.

The demands of this enforced creativity may generate concepts that do not meet the logical demands of existing mechanics. Here, said Foppl, was the deep reason for separating out technical mechanics as a special branch of knowledge—“diese Absonderung der technischen Mechanik als eines besonderen Zweiges der Wissenschaft” (11). Its practitioners must have the freedom to develop concepts of their own, and these might be distinct from those acceptable in the more reflective and leisurely branches of knowledge. For example, the application of hydrodynamic theory to turbines developed by Prasil and H. Lorenz, depended on certain artifices or tricks (Kunstgriffe) involving the idea of “forced accelerations.”53 The approach had been con­troversial, but Foppl defended it. He went on to say that in such cases subse­quent developments in science might permit a reconciliation. The anomalous concepts, special to engineering and technical mechanics, might be absorbed back into the main body of knowledge. But this was an open question, some­thing for the unspecified future rather than the urgent present. His main concern was to emphasize the restless force running through the scientific life of modern technology, which was, he said, like the life force in a tree that continually generated new branches.

In the peroration rounding off the introductory chapter, however, Foppl suddenly changed the metaphor. The life force of a tree was replaced by an­other kind of force. Knowledge was power, said Foppl, the power of a mod­ern technological state. The bucolic image was replaced by a military one. Those who first possessed the right theory (“die richtige Theorie”) might be able to intervene in nature at will. In this way, said Foppl, science, put at the disposal of humanity and its peoples, is the most powerful of all weapons— “und darum ist die Wissenschaft die gewaltigste Waffe, die Menschen und Volkern zu Gebote steht” (12).

What did Foppl understand by the “right” theory? His normative stan­dards were predictably active and pragmatic. Like many in his position in the technische Hochschulen, Foppl was fighting a war on two fronts. On one side were “humanistic” critics. These were usually outside the technical col­lege system, or only passing through it on their way to posts in universities. In as far as they wanted mathematics at all, they wanted it “pure.” On the other side were critics, often from within the technical colleges, who placed all the emphasis on practicality and were suspicious of any form of higher mathematics.54 These were the counterpart of the “practical men” in Britain who were so hostile to the work of the Advisory Committee for Aeronautics. In responding to the local, German, variant, Foppl dismissed such people as mere Praktiker.55 He had no time for them or their slogans about the conflict between theory and practice—“dem Gegensatze zwischen Theorie und Praxis” (3:vii.). For Foppl there was no such conflict:

Diese Behauptung lasse ich aber auf dem Gebiete der technischen Mechanik durchaus nicht gelten; hier kann nur von einem Gegensatze zwischen falscher oder unvollstandiger Theorie und der richtigen Theorie die Rede sein. Die richtige Theorie ist immer in Ubereinstimmung mit der Praxis. (3:vii)

I do not admit this claim as having any validity in the realm of technical me­chanics. Here one can only speak of the conflict between false or incomplete theories and the right theory. The right theory is always in agreement with practice.

Did Foppl mean that a theory was practical because it was right, or that it counted as right because it worked in practice? Taken in isolation, his wording was ambiguous. If, however, we recall Foppl’s insistence on the overpower­ing, practical necessities that dominate the life of the engineer—the “Zwang der Nothwendigkeit”—then the formal ambiguity can be resolved. In Foppl’s world, practice was the effective criterion not of an abstract and future truth but of acceptability and viability for the pressing moment. In the colleges of Cambridge, if Lamb is to be believed, the pursuit of truth had an aesthetic character. In the colleges of technology a theory was counted as right if, and only if, it worked.

Others in the field of applied mathematics in Germany may have made the case in different words, but Foppl’s general orientation toward engi­neering represented a widely held view. For example, in 1921 Richard von Mises started a new journal for applied mathematics—the Zeitschrift fur angewandte Mathematik und Mechanik. Prandtl and von Mises had been in correspondence after the war on new institutional arrangements for encour­aging applied mathematics. Prandtl mentioned that he and von Karman had been discussing the founding of a society to promote technical mechanics, “eine Vereinigung fur technische Mechanik,” and these exchanges were part of the process that culminated in the journal.56 On the first page of the new publication von Mises set out his conception of the task and goals of the dis­cipline and the role of the journal. He would have been conscious of stepping into a long-standing discussion about the role of mathematics in the German academic world but he had no desire to equivocate. He insisted that the core of the journal would be devoted to mechanics whose cultivation, he said, to­day lies almost exclusively in the hands of engineers, “deren Pflege heute fast ausschliefilich in den Handen der Ingenieure ruht.”57

Mathematics, said von Mises, covered a wide spectrum of activities so that the partition between pure and applied mathematics was a relative one, lo­cated differently by different practitioners. Each would count what was (so to speak) on their “left” as pure and what was on their “right” as applied. But there was not only this dimension to consider: the very content of mathemat­ics itself changed with time as new areas (for example, the concept of prob­ability) were brought within the scope of quantitative analysis. We must, said von Mises, accept this “two-fold relativity” in the identity of applied math­ematics. To overcome the definitional problems this created, he concluded that a practical, rather than a theoretical, specification of the field was called for. Applied mathematics and mechanics were to be defined as what was done, at that time, by scientifically oriented engineers. Thus,

Angesichts dieses Tatbestandes zweifacher Relativitat der Begriffsabgrenzung mussen wir nun eine praktische Erklarung dafur suchen, was wir hier im Fol – genden unter “Angewandter Mathematik” verstehen wollen. Es ist selbstver – standlich, dafi wir uns auf den Boden der Gegenwart stellen, und es sei hinzu – gefugt: auf den Standpunkt des wissenschaftlich arbeitenden Ingenieurs. (3)

Given the facts of this twofold relativity of the conceptual boundary, we must now seek for a practical explanation of what, in the following, we want to un­derstand by “applied mathematics.” It will be obvious that we take our stand on the basis of the present and, let it be added, on the standpoint of the scien­tific work of the engineer.

Clearly the two mathematical traditions that I have delineated had dif­ferent orientations: one more toward physics; the other more toward engi­neering. Obviously, Cambridge mathematical physics and German technical mechanics still had much in common. There were many respects in which they overlapped, and it was possible for results to be passed from the prac­titioners of one to those of the other. Prandtl’s early papers on elasticity and Foppl’s volume of the Vorlesungen devoted to the strength of materials were mentioned in Love’s treatise, while, in return, Foppl advised his more ad­vanced readers to consult Love’s work. Representatives of the two traditions attended the same conferences, even if this caused Lamb a touch of anxiety. Klein admired Cambridge pedagogy and tried, though without much suc­cess, to introduce it in Germany.58 Lamb’s Hydrodynamics was translated into German in 1907, again at Klein’s prompting, though later von Mises added a lengthy supplement to the book designed to build a bridge to the more tech­nical concerns and less formal orientation (“weniger formalen Richtung”) of German readers.59 Although G. H. Bryan evinced disdain for the intel­lectual level of the engineers in Joukowsky’s classes, he could write a respect­ful review of Foppl’s Vorlesungen in Nature saying, “Prof. Foppl’s treatises on technical mechanics are of a far more advanced character than the mechanics taught commonly to technical students in this country.”60 In his own way Bryan wanted to further the cause of applied mathematics in this country and was ready to hold up German efforts when it was expedient to do so. To this extent the acknowledgment of communality between British and German mathematical cultures was real enough.

Given this mixture of divergent tendencies and common ground, it is not surprising that the members of the respective traditions did not them­selves always have a clear awareness of the relations between them. This was epitomized in an exchange of letters that took place between G. I. Taylor and Ludwig Prandtl a number of years after the events described here. By the time the letters were written, in the 1930s, the magnitude of Prandtl’s contribu­tions had become known and widely admired. Taylor had written to say that he thought Prandtl deserved the Nobel Prize in physics. Prandtl’s response, in a letter of November 30, 1935, was not only becomingly modest but was also culturally revealing.61 He said that what he had done would not count as physics in Germany. Rather, it was a contribution to Mechanik.

Nach der in Deutschland ublichen Einteilung der Wissenschaften wenigstens wird die Mechanik heutzutage nicht mehr als ein Teil der Physik betrachtet, sondern steht als selbstandiges Gebiet zwischen der Mathematik und den Ingenieurwissenschaften.

At least according to the division of the sciences that is usual in Germany today, mechanics is no longer considered to be part of physics. Rather, it stands as an independent area between mathematics and the engineering sciences.

While Taylor now assimilated Prandtl’s work to physics, the Germans saw it as something distinct from physics and as standing between mathematics and engineering.62 The different stance toward engineering and its demands perhaps sheds light on why the circulation theory was actively resisted in Britain but accepted and developed in Germany. Before taking this argument further, however, I look at what Lanchester himself said to explain the rough ride given to his work. Lanchester’s account will give me an opportunity to look at another variable whose explanatory potential needs to be assessed, namely, the personalities of the main actors.

Knowing the predicted pressure distribution along a specified aerofoil opens up the possibility of subjecting the circulation theory of lift to a demand­ing empirical test. Does the predicted distribution correspond to the real

distribution in as far as it can be measured? In 1915, two years after Blumen – thal’s theoretical analysis, a detailed experimental study was published in the Zeitschrift fur Flugtechnik that was designed to answer this question. The pa­per was by Albert Betz, Prandtl’s close collaborator in Gottingen (fig. 6.10). It was called “Untersuchung einer Schukowskyschen Tragflache” (An investiga­tion of a Joukowsky wing).60

Betz used one of Blumenthal’s profiles and worked with a model wing that had a span of 50 cm and a chord of 20 cm. It had a curvature (f/l) of 1/10 and a thickness ration (8/l) of 1/20 and so corresponded exactly to the second of the four profiles described by Blumenthal (that is, the one shown in fig. 6.9). Betz’s aim was to use wind-tunnel data to test Blumenthal’s predicted lift and pressure distribution.

The model wing-section was manufactured from metal plate in the form of an airtight, hollow body and made to conform as precisely as possible to the theoretical, Joukowsky profile. Following Fuhrmann’s work on model airships, the wing was fitted with bore holes and the hollow interior was connected by a thin pipe leading from the wingtip to a manometer. This enabled pressure measurements to be taken at a number of points on the surface along the chord of the wing. Measurements were taken with one hole at a time exposed while the other holes were smoothly plugged. The line of bore holes was not positioned at the center of the span but was displaced a few centimeters to one side. This was to avoid interference to the flow of air over the holes from the strut that had to be attached to the wing in order to hold it rigidly in place in the wind tunnel. As well as the pressure, Betz also needed to know the overall lift and drag of the wing. For this the wing had to be suspended on a balance so that force measurements could be made. Dur­ing this phase of the experiment, the pipes leading to the manometer were disconnected and all the bore holes plugged.

It was necessary to make sure that the experimental arrangement pro­vided a good approximation to the infinite wing presupposed in the math­ematics. Joukowsky had simply made his wing section run from the top to the bottom of the shallow Moscow wind tunnel. This is the basis of all attempts to realize a two-dimensional flow, but Betz put a lot of effort into refining the technique. His aim was to make the test section as free as possible from disturbing effects produced by the walls of the tunnel and the join between the walls and the ends of the wing. An elaborate system of auxiliary sidewalls, gaskets, and seals was designed and tested to ensure a uniform flow across the experimental cross section of the wing. Once he had an acceptable approxi­mation to two-dimensional flow, Betz’s apparatus gave him two sets of data: (1) direct measurements of lift and drag and (2i) pressure measurements dis­tributed over the surface of the wing.

The direct measurements of lift and drag showed the familiar pattern, which partially conformed to, and partially violated, theoretical expectations. The observed lift increased in the predicted, linear fashion with angle of in­cidence, but only from about -9° up to about +10°, at which point the wing stalled. Even over this range the predicted lift was significantly higher than the observed lift. In fact, the observed lift was only about 75 percent of the predicted value. And, of course, there was an observed drag when, theoreti­cally, it should have been zero. There was also another general feature of the flow that Betz observed. Theoretically each angle of incidence should cor­respond to one, and only one, value of the lift. Betz found that if he took a sequence of readings in which the angle of incidence was increased in a stepwise fashion, and another sequence in which it was decreased, a given angle might correspond to one value of the lift in one sequence and another value in the other. There were two values of the lift corresponding to each of these angles, not one. This effect was particularly noticeable above the stalling angle. Thus, at +15°, the coefficient of lift had the values of 0.68 and 0.55. Such a phenomenon fell wholly outside the scope of the theory.

The most important results, however, were those relating to the distri­bution of pressure. Here Betz’s graphs of the manometer readings showed a definite similarity to the theoretical graphs prepared by Blumenthal and his colleagues at Aachen. Betz’s results are shown in figure 6.11. Note that

Betz used a convention different than Blumenthal’s when drawing his dia­grams, and the data for the upper side of the wing are now placed below the base line. Significantly, the general shape of the graph derived from theory and that of the graph derived from experiment were the same. Betz, how­ever, pressed the comparison into greater detail. He was interested in getting information about the residual deviations between theory and reality, “die Abweichungen der Theorie von der Wirklichkeit” (173). This was the stated purpose of the experiment. He therefore drew attention to where the empiri­cal distribution differed from the theoretical distribution. The areas under the empirical graphs were clearly not the same as those under the theoretical graphs. These areas were proportional to the lift, and the theoretical area was significantly greater than the observed area. This was consistent with the fact, mentioned earlier, that the directly measured lift was less than the theoreti­cally predicted lift. What was the cause of the difference, and what should be done in response to it?

Like Fuhrmann, Betz located the source of the difference between the the­oretical and empirical flow in the tail region. Theoretically, if the Kutta condi­tion is satisfied and the wing profile is that of a pure Joukowsky contour, and if the air acts like an ideal fluid, then the flow along the upper surface will meet the flow along the lower surface in a smooth way at the trailing edge. In reality, however, the air did not behave in this way at the trailing edge, so Betz made a conjecture. It was a characteristically Gottingen conjecture (see fig. 6.12).

Betz suggested that, although the flow of air along the lower surface runs smoothly along the common tangent, the flow along the upper surface does not. Rather, it detaches from the upper surface before reaching the trailing edge, and this leaves a gap between the two flows. The intervening space be­tween the flows, said Betz, constitutes a turbulent wake filled with “Karman vortices” (177).

Betz argued that the effect of this separation is twofold. First, it disrupts the pressure relations in the vicinity of the trailing edge and disturbs the equi­librium between the forward-pointing and backward-pointing components of the pressure distribution. Since it is this equilibrium that generates the zero drag of an (ideally) efficient aerofoil, the disturbance must be a contributory cause of the observed drag. Second, the vortices in the wake draw off energy, and this has the effect of lowering the circulation around the aerofoil and hence diminishes the lift. Betz conceded that it was difficult to analyze these processes rigorously but suggested a simple (and intriguing) way to model the situation using the resources of inviscid theory. He proposed rejecting the Kutta condition and relocating the stagnation point. Whereas Kutta had used the position of the stagnation point to fix the amount of circulation, Betz reversed the process. He used the amount of circulation to fix the stag­nation point.

Betz began with the value for the lift at a given angle of attack that he had found in his experiment. He then inserted the value into the basic Kutta-

Joukowsky theorem L = p V Г. Because he knew the density and velocity of air in the wind tunnel, the formula allowed him to deduce the value of the circulation. He then used this empirical value of the circulation to tell him where the rear stagnation point must be relocated according to the theory of inviscid flow. (Betz was working with a Joukowsky profile, so this point could be calculated by transforming the flow around a circular cylinder.) Given that the empirical value of the circulation is lower than the theoretical value, the stagnation point is on the top surface of the aerofoil, rather than precisely at the trailing edge. It was then possible to replot the theoretical speed and pres­sure distributions and compare them afresh with the empirical curves. The question was whether the revised location of the stagnation point brought the empirical and theoretical graphs onto closer accord. The method automati­cally equalizes the areas under the empirical and theoretical curves but the question remains: do the distributions agree? Betz’s revised graph, superim­posed on the empirical curve, is shown in figure 6.13. The improvement is clear. The graphs are now almost identical. Betz pronounced the agreement to be “extraordinarily good.” As he put it,

Sehen wir von der nachsten Umgebung der Hinterkante ab, fur die ja Voraus – setzungen der theoretischen Stroming vollstandig andere sind wie in Wirk – lichkeit, so mussen wir die Ubereinstimmung der beiden Kurven aufieror – dentlich gut bezeichnen. (177)

If we disregard the vicinity of the rear edge, where indeed the presuppositions of the theoretical flow wholly differ from reality, then we would have to char­acterize the agreement of the two curves as extraordinarily good.

Betz had thus brought theory and experiment into closer accord. His proce­dure involved interweaving them in an interesting way, and the methodologi­cal principles implicit in the process are worth looking at with some care.

The Kutta condition had become established as one of the central as­sumptions of the circulation theory. Could Betz really afford to modify its role in this way? The overriding advantage of the Kutta condition was that it avoided the need for the ideal fluid to move around the trailing edge at an in­finite, and physically impossible, speed. How did Betz justify the reintroduc­tion of infinite speeds given that the need to avoid them was routinely cited whenever the Kutta condition was invoked? The point was addressed explic­itly in the paper. The argument was as follows: Since perfect fluid theory is known to be unrealistic from the outset, said Betz, one more piece of unreal­ity hardly matters. The entire approach is an artifice, so this should not be disturbing. The infinite speeds are just the way that the complicated, physical

processes at the trailing edge receive some manner of recognition within the terms of the theory. Their presence in the analysis simply indicates that fur­ther assumptions need to be introduced to mediate between reality and the idealized picture. Outside the wake, the flow can be reasonably modeled by ideal-fluid theory, and the presence of the wake can be taken into account by lowering the value of the circulation. This approach can be seen as the first step toward a better account of the phenomenon. As Betz put it,

Dafi dabei ein Umstromen der scharfen Hinterkante stattfinden mufite, was praktisch unmoglich ist, braucht uns nicht zu storen, da ja die Stromungen. . . bis zu dem gemeinsamen Ablosungspunkt nur ein theoretischer Ersatz sind fur die in Wirklichkeit vorhandenene Wirbelbewegung. (177)

That this would have to result in a physically impossible flow round the sharp trailing edge need not disturb us. This is because the flow. . . up to the com­mon point of separation is only a theoretical substitute for the vortex motions that exist in reality.

The assumptions behind this talk of a “theoretical substitute” (“theoretischer Ersatz”) can be clarified by noting what Betz had said about d’Alembert’s paradox. Notoriously, the theory developed by Kutta and Joukowsky pre­dicted that a wing will have zero resistance. Betz, however, defended the use of an inviscid theory as an approximation, “even though it does not permit any statements to be made about the resistance” (“trotzdem sie uber den Widerstand nichts auszusagen vermag”; 173). What did Betz mean by this? Surely, the theory does permit a statement to be made about resistance. In­deed, it requires that a statement should be made: namely, the false statement that the resistance is zero. Betz knew this, so what he must have meant was that the theory does not permit any useful statement to be made. The theory doesn’t shed any light on the resistance. His question was: To what practical purpose can the theory be put? The theory was being viewed as a tool rather than a body of propositions. Perfect fluid theory is a useful tool for certain purposes but not for others. Betz was telling his readers that questions about the utility of the theory, rather than its literal truth, should be uppermost in their minds. That is why they should not be unduly disturbed by theoretical deductions that entail infinite speeds.

A number of significant changes, both organizational and personal, took place in the higher reaches of British aeronautics at the end of the war. The size of the aeronautical section at the National Physical Laboratory had grown considerably during the conflict. Starting from three or four active workers in 1909, the section had expanded to around forty by the time of the armistice.39 Predictably, the return of peace meant that the budget was now to be cut back. Lord Rayleigh had died in 1919, and the Advisory Committee he had guided for a decade was formally dissolved and reconstituted as the Aeronautical Research Committee (ARC). The new committee held its first meeting on May 11, 1920.40 Glazebrook was given the job of restructuring it and preparing it for its new peacetime role. The National Physical Laboratory and the Aero­nautical Research Committee now came under the aegis of the newly formed Department of Scientific and Industrial Research (DSIR).41 Horace Lamb had been appointed to the Aerodynamics Sub-Committee in July 1918 and later joined the full committee.42 In an attempt to avoid the old hostility between the scientists and the manufacturers, there were now to be representatives of industry on the committee. J. D. North, of Boulton Paul, was appointed to the Aerodynamics Sub-Committee to represent the Society of British Aircraft Constructors. Bairstow left the National Physical Laboratory in 1917 and took up a post with the Air Board, the precursor to the Air Ministry, though he continued to serve on the new committee.43 Bairstow then moved again and become the Zaharoff Professor of Aviation at Imperial College, London. Sir Basil Zaharoff, who financed the chair, was an international arms dealer.44 Shortly afterward Emile Mond provided the money to set up a chair in aero­nautics at Cambridge in memory of his son killed flying on the western front. This chair was taken by Melvill Jones.45 Bairstow’s post as superintendent of the Aerodynamics Department at the NPL was taken over by Southwell, who moved from Farnborough to Teddington. Lanchester, who sometimes felt that Rayleigh was the only sympathetic member of the committee, left a year after Rayleigh’s death. Lanchester had been assiduous in his duties but had al­ready resigned from the Aerodynamics Sub-Committee in December 1918.46 Now that the emergency of the war was over he felt able to cite pressure of work as a basis for leaving. In his letter to the chairman of the Aerodynamics Sub-Committee he expressed “great pleasure in having been able to serve the committee,” but the retrospective account he gave of his departure from the full committee was very different in tone. He complained that he had been sidelined, snubbed, and deliberately edged out by Glazebrook.47

At the moment that Lanchester left the Whitehall scene feeling, justi­fiably, that his ideas had been ignored, moves were under way that would eventually lead to the triumph of the circulation theory he had pioneered. Two things happened. First, on November 13, 1920, Southwell received a let­ter from Prandtl, who sent him some up-to-date papers on wing theory and material from the Technische Berichte. Prandtl explained that his action had been prompted by his meeting with William Knight. Knight had apparently told Prandtl that Southwell wanted to get hold of information about develop­ments at Gottingen. Southwell replied on November 29 with thanks and ten­tatively asked Prandtl for details about his wind tunnel and the techniques for keeping the flow steady. He also stressed that the exchange with Prandtl had to be considered personal rather than official because of the British govern­ment’s policy of restricting formal contact with German institutions. Prandtl sent Southwell the required data about the air flow and said that more in­formation would soon be published in a volume to be titled Ergebnisse der Aerodynamischen Versuchsanstalt zu Gottingen.48

The second development was that two Farnborough scientists, Robert McKinnon Wood and Hermann Glauert, both members of the Chudleigh

set, were sent to Germany to report on the situation. McKinnon Wood, a product of the Cambridge Mechanical Sciences Tripos, was deputy director of the Aerodynamics Department at Farnborough and worked on propel­lers and the experimental side of aerodynamics.49 Hermann Glauert (fig. 8.3) had studied mathematics at Trinity College, Cambridge, where his circle of friends included David Pinsent, G. P. Thomson, and Ludwig Wittgenstein.50 He graduated with distinction in the first class of part II of the Tripos in 1913 and won an Isaac Newton studentship in 1914 and the Rayleigh Prize for mathematics in 1915.51 Glauert was born in Sheffield. His mother was an Englishwoman who had been born in Germany, while his father, a cutlery manufacturer, was German but had taken British citizenship. Originally spe­cializing in astronomy, Glauert published a number of papers on astronomi­cal topics at the beginning of the war and then, through a chance meeting with W. S. Farren, he was appointed to the staff of the Royal Aircraft Factory in 1916.52

Based in the Hotel Hessler in Charlottenburg, Glauert wrote on Janu­ary 21, 1921, to make contact with Prandtl and ask if was possible to arrange a

visit to Gottingen.53 The approach could not have been more different from Knight’s. Knight had sent a typed letter, in English, on elaborately headed NACA notepaper. The letter was replete with office reference numbers and subject headings and was introduced with a flourish of (questionable) diplo­matic credentials.54 Glauert penned his note in German on a modest sheet of unheaded paper. He introduced himself as a fellow of Trinity who worked at Farnborough and explained that he was very interested in the reports shown to him by his friend Herr Southwell. Could he and his friend Herr Wood, also of the Royal Aircraft Factory, come along next Monday? The visit duly took place but cannot have been an extended one because on February 2 Glauert was writing from Farnborough (again in German) to say that he and Wood were safely home after a thirty-six hour journey. All of the technical material that Prandtl had given them, he reported, had been carried over the border without difficulty. In return Glauert sent Prandtl a copy of Bairstow’s new Applied Aerodynamics.55 It was, he said, currently the best English book on aerodynamics.

Bairstow’s Applied Aerodynamics, published in 1920, offered a massive com­pilation of design data from the aerodynamic laboratories which, for Bair – stow, primarily meant from the National Physical Laboratory. It was com­prehensive and detailed but, as far as lift and drag were concerned, heavily empirical. The circulation theory of lift was placed on a par with the dis­continuity theory and rapidly dismissed. Both theories were said to be based on “special assumptions,” that is, ad hoc devices designed to get around the fatal zero-drag result. Kutta’s work, according to Bairstow, offered no more than a “somewhat complex and not very accurate empirical formula” (364). No account, he complained, was given by Kutta or Joukowsky of the critical angle of stall. Bairstow admitted that Joukowsky had found a way to avoid the infinite speeds at the leading edge of a wing profile, but he spoke of the Joukowsky transformation as if it were little more than a mathematical trick. Bairstow called it a “particular piece of analysis” and did not deem it suf­ficiently important to explain it to the reader. Prandtl must have perused Glauert’s gift with mixed feelings. He would have agreed with all of Bairstow’s facts but none of his evaluations. In particular, he would surely have dissented from Bairstow’s conclusion that it “appears to be fundamentally impossible to represent the motion of a real fluid accurately by any theory relating to an inviscid fluid” (361). Wasn’t this exactly what Prandtl and his colleagues had just done for the flow of air over a wing?

It is clear from the subsequent letters exchanged between Glauert and Prandtl that, during the visit, their conversations had been confined to tech­nical matters. Prandtl was now keen to discuss politics as well as aerodynam­ics. He was distressed by the economic and political situation, particularly the stance of the French and the severe reparations that were being demanded. Glauert expressed agreement with much that Prandtl said on these topics, for example, with the “absurd restrictions that have been placed on the develop­ment of German aviation,” but in his replies he encouraged Prandtl to see things in a less pessimistic light. Glauert explained that not everyone in Brit­ain agreed with these policies, and he thought there were strong economic reasons why, sooner or later, they would be modified. Writing now in English, he drew Prandtl’s attention to the influential arguments of the Cambridge economist John Maynard Keynes and mentioned that opposition to punitive sanctions was also part of the official policy of the Labour party.56 He even in­cluded a reassuring cutting from the correspondence columns of the Times.57 The concern was not just personal; it was also professional. Glauert was wor­ried that Prandtl would become so antagonized by allied policy that he would cease to take part in scientific exchanges. The anxiety was more than justified. The academic atmosphere in the immediate postwar years was a poisonous mixture of bitterness, intransigence, boycott, and counterboycott.58

The immediate result of the Glauert-Wood visit to Germany was the production of two confidential reports. In February 1921 McKinnon Wood produced Technical Report T. 1556, “The Aerodynamics Laboratory at Gottingen.”59 He argued (contrary to government policy) that Gottingen should be included in any future international trials that were envisaged to clarify the discrepancies that existed between the results of different labo­ratories.60 When the results of the British and Germans were compared, McKinnon Wood noted that the Gottingen channel gave the same lift and drag, but always for an angle of incidence smaller by about one degree. He also studied and reported on the complicated “three-moment” balance mechanism used to measure the aerodynamic forces. A blueprint of the bal­ance was included in the report. He judged that the Gottingen balances were “very inferior” in sensitivity to the British but then conceded that “our bal­ances and manometers are unnecessarily sensitive for most of the work for which they are required” (14). McKinnon Wood concluded by saying that “Mr. Glauert discussed Prandtl’s aerofoil theories with him and obtained some further papers. A discussion of these will be embodied in a separate paper (T. 1563) which Mr. Glauert is writing” (21). The “discussion” to which McKinnon Wood alluded turned out to be a piece of brilliant advocacy that was undoubtedly a major factor in undermining resistance to the circulation theory in Britain. Glauert had a gift for clear exposition and for seizing the essentials of the subject. Once he had accepted that Prandtl’s theory repre­sented the path to follow, Glauert produced a notable series of papers and reports explaining, testing, and developing the achievements of the Gottin­gen school. He corrected inadequate formulations and produced important extensions of the theory, as well as confronting the skeptics.

What made Glauert special? Why did he, with his impeccable Tripos background, strike out in a direction that had hitherto been unattractive to experts who shared with Glauert the intellectual culture of the “Cambridge school”? The “practical men” had always been divided over Lanchester’s the­ory of lift, but the “mathematicians” had been unanimous in their skepti­cism. Why did Glauert break ranks? No definitive answer can be given to this question, though one fact stands out and invites speculation. The Ger­man name, the German father, the command of the German language may have generated some affinity with the body of German work that was under consideration. These facts distinguished Glauert from British experts such as Bairstow who did not have the command of German that would have enabled them to read the literature or converse with Prandtl.61 (At that time Prandtl had little knowledge of English.) Of course, given the bitterness of the war, personal links to Germany might have had quite a different effect. Such links could be sources of difficulty, and there is evidence that they caused prob­lems, and some inner turmoil, for Glauert. Referring to the outbreak of war in 1914, Farren and Tizard said of Glauert: “His German descent was an em­barrassment to him, and he wisely decided to stay where such trivial matters did not assume the importance that they did elsewhere, and where he could work in peaceful surroundings, though not with a peaceful mind. His friends were far afield, and as time went on he became more and more restless and concerned with the difficulty of his position.”62

Some people in Glauert’s position would have kept their distance from all things German, and even shed their German name.63 One might specu­late that, in Glauert’s case, the balance in favor of the circulation theory was tipped by the opportunity to meet members of the Gottingen group. His visit to Germany enabled him to explore the mathematics of the circulation theory with Prandtl face-to-face. Others had visited Gottingen before the war, but British experts did not have a great deal of direct contact with their Ger­man counterparts.64 Even here it is necessary to be cautious about the im­pact of personal contact. Glauert was impressed by the circulation theory before he went to Germany. Doubts and qualifications dropped away after the visit to Gottingen and his advocacy became more confident, but he had begun to explore the theory through what he read in Southwell’s copies of the Technische Berichte. Farren and Tizard suggest that exposure to engineer’s shoptalk in the Chudleigh mess at Farnborough during the war made Glauert sympathetic to the needs of engineers and hence (one may suppose) to the theoretical approaches adopted by the engineers. Beyond this, little can be ventured in terms of explanation. It must simply be accepted that, equipped with the recent papers, Glauert made it his business to explore the Gottingen theory in great detail. He became committed to it, even when this led him to diverge from such authorities as Leonard Bairstow, Horace Lamb, R. V. Southwell, and G. I. Taylor.65

Glauert would have known that, however cogent he made his book, he could not meet all the demands of his intended audience. The diversity of interests that would inform the response would pull in opposing directions. The struc­tural tensions, present in British aerodynamics from the outset, were still at work. By its very nature, Glauert’s Elements of Aerofoil and Airscrew Theory could not satisfy the prejudices of both the practical engineer and the math­ematical physicist. Indeed, the book was designed to bring about a change of approach in both parties. Until it had worked its effect it was bound to be viewed with a certain reservation from both sides, even if, at the same time, its virtues were acknowledged.

The review that appeared in the Journal of the Royal Aeronautical Society was signed “A. R.L.” With its mixture of bluff praise and barbed comment, the review was such that no regular reader would have failed to recognize it as the work of Major A. R. Low.56 Glauert was identified as one (but only one) of the leading exponents of the Lanchester-Prandtl approach, and the Ele­ments was welcomed as (perhaps) the first full-length book on the subject in the English language. There was the hint that engineers might find Glauert’s discussion too advanced, and Low strongly recommended that students read a work by H. M. Martin “as an introduction to the volume under review, and, as well, for Mr. Martin’s mastery of elementary exposition” (167).57 Low simultaneously praised Glauert for the adequacy of his general references, which would “introduce the reader to the most important German work,” and criticized him for not citing Fuchs and Hopf’s Aerodynamik of 1922, which “quite evidently influenced the author, both as to selection and arrangement of materials” (168). The book by Fuchs and Hopf was broader in scope than the Elements, covering both lift and stability, and Low had reviewed it in the Aeroplane. While he had praised their treatment of lift, he had been scathing about their treatment of stability.58 Low acknowledged Glauert’s originality in using the method of images to arrive at a formula for tunnel interference effects in the case of rectangular (as distinct from circular) tunnels but quib­bled at an “unnecessary reference to Hobson’s Trigonometry” (168) to deal with a mathematical point that Low considered elementary.59 Low concluded his review by describing the Elements as “an important contribution to Eng­lish aerodynamic literature” and (high praise indeed) as a book that “should be of the greatest value to all designers of aircraft” (168).

Approaching the Elements from the direction of the mathematician rather than the engineer, R. V. Southwell reviewed it for the Mathematical Gazette.60 He praised Glauert’s “power of concise exposition” (394) and said the book gave “an admirable account of a fascinating theory.” It could be recommended as “indispensable to every student of modern aerodynamics” (395). But, as well as offering praise, it is clear that Southwell was taking care to locate Glauert’s achievement in a particular way. He began by noting that when Mr. Asquith first appointed the Advisory Committee for Aeronautics in 1909, “nothing seemed more certain than that aerodynamics must develop

as a purely empirical science” (394).61 Theoretical hydrodynamics was not sufficiently developed to take account of both inertial and viscous forces. Today, said Southwell, that “difficulty is not yet overcome, but it has been turned” (394). Prandtl’s wing theory is not “an exact theory,” but Prandtl “has supplied what for practice is almost as useful—a theory which can pre­dict” (394).

Southwell then clarified his distinction between exact and predictive theories. Consider Glauert’s chapter 8, which contained an account of skin friction and the origins of viscous drag. Southwell granted that Glauert’s brief treatment was appropriate given the limited purposes of the Elements but ex­pressed the hope that Glauert would produce a follow-up volume to expand the “somewhat slender outline” of the present chapter. The follow-up vol­ume would call for a “slightly altered arrangement” of the material. It appears to me, said Southwell, that

we ought to recognise not one “Prandtl theory,” but two. The first forms the main subject of the present work; its methods are those of the classical theory, and its assumptions, based on Lanchester’s picture of the flow pattern, are justified, ultimately, by its success in prediction. The second, which develops the notion of the “boundary layer” is more fundamental, more difficult, and (probably) less productive of concrete results than the first; its aim is to ex­plain the circulation round a lifting wing in terms of the known equations of viscous flow. (395)

The implication was that Glauert had adopted an “arrangement” of his material that did not adequately recognize the difference between the two theories. Glauert ran these two distinct theories together and aspired to a “combined” presentation. This may help the student, said Southwell, but it cannot do justice to either theory because “their methods are too distinct to permit a really satisfactory blend” (395). He did not believe that they could be combined in the way that Glauert wanted. “For the combined theory seeks to bring phenomena, in their very essence dependent on the viscosity of the fluid and its interaction with the solid boundary, within the scope of analysis which he knows is strictly applicable only to vortex motions existent through­out all time in a fluid devoid of all viscosity” (395). Southwell thus insisted on keeping apart what Glauert had sought to bring together. What the author had aspired to unify, the reviewer saw as incompatible. The themes invoked in Southwell’s review were familiar and characteristic of the British experts: there was the desire for a “fundamental” theory based on Stokes’ equations, a commitment to the “essential” difference between real and perfect fluids, and the appeal to the eternal character of vortices in a perfect fluid, that is, to Kel­

vin’s theorem. That Glauert, like Prandtl, was deliberately trying to overcome the idea that there is an “essential” difference between real and perfect fluids finds no recognition. Southwell acknowledged in Glauert’s unified presenta­tion not a principled methodological stance but a mere pedagogical expedi­ent, an “arrangement” of material to help students—and an arrangement that could not be sustained in the face of reality or in the pages of a more advanced treatise.

Southwell said that the views he expressed “imply no criticism” of Glau – ert’s book. The claim may look disingenuous but I think it should be accepted as authentic. The words would make sense if Southwell were reading Glau­ert’s book as an exercise in technology rather than physics. Once Prandtl’s wing theory was understood as no more than an instrument of prediction, as something that could be assessed using purely pragmatic criteria, then the real business of science could be thought of as proceeding in parallel to the technology. There would be no need for any quarrel between those engaged in the two distinct sorts of activity, provided they were kept apart and not confused with one another. Thus Southwell could honestly declare that he was not criticizing Glauert’s book but simply making it clear what manner of book it was, and what criteria were appropriate for its assessment.

This left just “one small detail” (395) that Southwell certainly wanted to criticize in an explicit way. He was worried about the imaginary roller bear­ings that Glauert interposed between a fluid and a material body or between two layers of fluid moving in different directions or with different speeds. References to roller bearings cropped up at a number of points in Glauert’s book, for example, on pages 95, 100, and 117, and were represented diagram­matically on page 131. Southwell thought such talk was misguided, and he implied that Glauert should know better. Vortices don’t behave like roller bearings, and it won’t help the beginner to understand “the purely math­ematical concept of vorticity” (395), that is, the technical definition of the rotation of a fluid element. Southwell’s point was that “vorticity,” as the term is used in fluid dynamics, can be present when nothing in the flow behaves like a “vortex,” as that term is used in common language, that is, nothing is swirling, rolling, or rotating. For example, mathematically, “vorticity” is pres­ent when two immediately adjacent layers of ideal fluid move horizontally with uniform but different speeds. All the fluid in the respective layers moves in straight lines, but for the mathematician, this phenomenon is equivalent to an infinitely thin sheet of vorticity between the layers. Talk of “roller bear­ings,” however, will produce an incorrect picture in the mind. The begin­ner “will misunderstand either the vortex sheet, or the action of roller bear­ings” (395). The harshest criticism was thus directed at Glauert’s engineering imagery. Southwell was not, in general, against visualization.62 The complaint was against the way that viscous processes and the viscous boundary layer were represented in nonviscous terms.63

Despite these reservations, the publication of Glauert’s Elements in 1926 represented the de facto victory of the circulation theory of lift among Brit­ish experts. The theory and references to Glauert’s exemplary account of it found their way into all subsequent treatises and textbooks, such as Lamb’s Hydrodynamics and Ramsey’s Treatise on Hydromechanics. The victory was, of course, underpinned by the steady accumulation of evidence from experi­mentalists such as Fage.64 The increasingly secure position of the circulation theory was, however, of a qualified kind. The victory was no simple rout of the opposition. The situation might be described with the use of political metaphors by saying that territory was conceded and new spheres of influ­ence agreed on. The power of the circulation theory had been demonstrated, and a certain zone of occupation was now recognized—though not the full legitimacy of what had taken place. The task now was to get on with life under the new dispensation. In the Great War, Germany may not have prevailed, but in the field of practical aerodynamics a new respect was accorded to the circulation theory and Prandtl’s wing theory. In 1927 Prandtl was invited to London to deliver the Wright Memorial Lecture to the Royal Aeronautical Society and to receive the Gold Medal of the society.65

There had been a previous suggestion that Prandtl might give a talk, which had been conveyed via Glauert in 1922. Prandtl had felt compelled to turn down the invitation, however, because of his lack of English.66 The Wright Lecture was a much grander affair, and Prandtl, who clearly appreciated the invitation, now felt better equipped to cope, though he still had some anxie­ties. In the preparatory exchange of letters with the chairman and the secre­tary of the society he fussed over what he should wear. Should he be in Frack, that is, tailed coat? In hesitant English he announced that “I have at this time English lessons and believe to be able up to the date of the lecture, to read the paper myself.”67 In the event, despite displaying the recommended tails, white tie, and white waistcoat, he only delivered the opening passages of the lecture and then called on the help of Major Low. Low, who had worked with Prandtl to translate the text, read the remainder.68

Those opening passages, however, touched on a matter of some delicacy. They concerned the origin of the theory of the aerofoil and the relative con­tributions of Prandtl and Lanchester. Who invented the theory and who should get the credit? Prandtl was diplomatic but forthright. He said that Lanchester had worked on the subject before he, Prandtl, had turned his at­tention to it and that Lanchester had independently obtained an important part of the theory. Prandtl insisted, however, that the ideas he used to build up his theory had occurred to him before he read Lanchester’s 1907 book. This prior understanding, he argued, may explain why “we in Germany were better able to understand Lanchester’s book when it appeared than you in England” (721). The truth, Prandtl went on, is that “Lanchester’s treatment is difficult to follow.” It makes “a very great demand on the reader’s intuitive perceptions,” and “only because we had been working on similar lines were we able to grasp Lanchester’s meaning at once” (721).

Is Prandtl here corroborating Glazebrook’s excuse for the British neglect of Lanchester? Surely not, though he certainly shared some of Glazebrook’s ideas about Lanchester’s work. Like Glazebrook, Prandtl did not countenance the possibility that it was the understanding of Lanchester, rather than the failure to understand him, that lay behind the British response. But, while go­ing along with part of Glazebrook’s story, Prandtl’s comments actually serve to accentuate the tensions between the different parts of Glazebrook’s excuse. They made it even more necessary to explain why the Germans were in a po­sition to grasp Lanchester’s meaning when, allegedly, the British had not been able to rise to the occasion. Glazebrook had excused one failure by citing another failure, and what Prandtl had to say aggravated rather than alleviated this logical weakness.69

My example of trailing vortices depended for its force on the difference be­tween the understanding of two groups of agents, the scientists and the pilots—the one group believing that trailing vortices had no practical sig­nificance, the other group knowing in a tacit and practical way that they did. What happens to the arguments for relativism when all parties know the same thing? This question is important because the universality of scientific under­standing is often taken to provide an adequate response to relativism. There is only one real world; the laws of nature are the same in London and Berlin; a true theory applies everywhere, and science knows no bounds of nation or race. “It is transnational and, despite what sociologists claim, independent of cultural milieu.”86 If science is independent of cultural milieu, then it cannot be relative to cultural milieu. Granted the premise, the conclusion follows, but my case study shows that the premise is false. The understanding of the phenomenon of lift was not the same in London and Berlin or Cambridge and Gottingen.

Such a response, based upon mere historical fact, is unlikely to satisfy the critics of relativism. It will be said that my study deals with a passing phase. Isn’t the important thing what happened after the episode that I described— when the truth emerged? The transnational character of science may take time to reveal itself, and progress may be inhibited by unfavorable social con­ditions, but universality triumphs in the end. It will be insufficient for the relativist to object that the antirelativist has shifted the discussion from what was the case to what ought to be the case or to what will be the case. The pic­ture of universal knowledge has force because there is, here and now, much science that is indeed transnational. This fact cannot be denied, so what can the relativist say?

Frank argued that relativism is consistent with universality. He said that the conditions leading to the spread of scientific knowledge were the very ones that ought to encourage a healthy relativism. As experience is broad­ened, the tendency to treat a belief as absolute will be undermined. Dogmati­cally held theories will encounter challenges, and a growing appreciation of the complexity of the world will undermine their apparently absolute status. Absolutism is parochialism—the cognitive equivalent of parish-pump pa­triotism. But the central reason why universalism is no threat to relativism is that the extent of a cultural milieu is purely contingent. In principle, a culture could be worldwide. The universal acceptance of a body of knowledge could only serve as a counterexample to relativism if universality indicates, or re­quires, absolute truth.

Let me explain this by an example. By the early 1930s Hermann Glauert had become a fellow of the Royal Society and the head of the Aerodynamics Department at Farnborough. He was at the height of his powers and had just finished a lengthy contribution on the theory of the propeller for William Durand’s multivolume synthesis of modern aerodynamic knowledge. Then tragedy struck. On August 4, 1934, a Saturday, Glauert took his three chil­dren for a walk across Laffan’s plain near Farnborough. The party stopped to watch some soldiers who were arranging an explosive charge to blow up a tree stump. The party stood, as required, at a safe distance some two hundred feet away, but the instructions they had been given were based on a misjudg – ment. Glauert was struck by a piece of debris from the explosion. No one else was hit, and his children were unhurt, but Glauert died instantly.87 In a dignified letter to Theodore von Karman, who had now moved from Aachen to the California Institute of Technology, Glauert’s wife (Muriel Barker) re­called the last time she and her husband had met von Karman. Along with G. I. Taylor they had all sat together, in the garden of Taylor’s Cambridge house, having tea and making plans for the future.88 Von Karman replied, in English: “The few people really interested in theoretical aerodynamics always felt as one family, and I am very proud to say that I had the feeling that your late husband and I were really friends, also beyond the common scientific interests.”89 Von Karman’s metaphor of the “family” to describe the relation­ship between leading members of the profession is striking. It resonates with, and lends support to, the theme of the transnational or universal character of science, though of course allowance must be made for the circumstances in which the expression was used. Perhaps it is the exchange of letters itself, rather than any particular choice of words, that should be considered the salient point. Former enemies in a bitter war are now consoling one another and affirming their solidarity. This epitomizes the increasingly cosmopolitan nature of the scientist’s world, at least as it was emerging, in the interwar years, in the field of aerodynamics.90

How is this emergence of a transnational science to be interpreted? There is a methodological choice to be made. In framing a response the choice lies between (1) invoking some form of inner necessity governing scientific progress and (2) settling for mere contingency. On the first approach it will be tacitly supposed that a “natural tendency” or telos is at work guiding the development. This idea will not recommend itself to an empirically minded analyst, who would therefore choose the second approach. Internationalism is to be analyzed strategically, not teleologically. The relevant comparisons are with the globalization of markets or the spread of the arms race.

Any move toward transnational knowledge should be interpreted in a wholly matter-of-fact manner. Sometimes scientists will reach out across na­tional boundaries, and sometimes they will not. It will depend on opportuni­ties and on perceived advantages and disadvantages and will vary with time and circumstance. (Recall Prandtl’s ambivalent reaction to cooperation in the immediate postwar years.) There will be no inner necessity at work, and references to the “transnational” character of science should not be accompa­nied by starry-eyed sentiments about Universal Truth. Why was von Karman in the United States? What was he doing at Caltech, and who was support­ing his research? 91 Each case needs to be examined by the historian for its particular features and causal structure. Thus in my study I found there was a phase when the reports of German work prepared for the Advisory Com­mittee for Aeronautics lay gathering dust, and there was another phase when copies of the Technische Berichte were sought with urgency. Both should be counted as equally natural. The universality of science and technology, or the absence of universality, depends on familiar, human realities. Some of these will be the brutal realities of war, power politics, and military and diplomatic strategy. Others will be the softer and more agreeable realities of the kind recalled in the exchange between Glauert’s young widow and von Karman— such as taking tea on a Cambridge lawn. These two levels, so different and yet so intimately connected, need to be brought together and linked to the calculations and experiments carried out at the research front. This is what I have sought to do.92

I now move from the context to the content of the Advisory Committee’s work to see how it carried out the research program it had originally set itself. Mr. Asquith assured the House of Commons on May 20, 1909, that the new committee would pursue the problems of aeronautics “by the application of both theoretical and experimental methods of research.”83 No significance

should be attached to the word order, placing theory before experiment, be­cause both found vigorous expression, although the relation between theory and experiment assumed very different forms in the different areas of the committee’s work.

Important tests on full-scale aircraft were carried out at Farnborough, but the main arena in which theory and experiment confronted one another was the wind channel (and sometimes the water channel) in which fluid flow over model wings and model aircraft could be observed and measured. The National Physical Laboratory already had a small water channel, and even a small vertical air channel, but the first task of the ACA was to oversee the construction of a better and more modern horizontal air channel to match those already known to be in use in Paris and Gottingen. By the end of the first year they were able to report on their plans to build a 4 X 4 X 20-foot channel with a draught of nearly 50 feet per second produced by a fan of 6 feet in diameter.84

Difficulty was experienced getting a steady flow, but by keeping the veloc­ity down to 30 feet per second, the flow was found to be “satisfactorily uni­form.” The measuring apparatus for registering the aerodynamic forces on various plates and models was also ready. It was now possible to measure the force component perpendicular to the flow (the lift) and that in the direction of the air current (the “drag” or “drift”). The apparatus could also be set up to determine centers of pressure, and the model could be adjusted to be at any angle with the current without stopping the flow.85

How was the apparatus to be used? Would it be employed to study the behavior of wings and other models in a purely empirical manner to build up an inductive knowledge of the regularities in their behavior? Or would it be used in a theory-testing manner for work that started not with the observable facts but with some theoretical conjecture? If the latter, what theories would be tested and where would they be found? The answer is that both strategies were present in the empirical work. Many of the measurements on model wings involved the highly empirical, and essentially inductive, engineering method of “parameter variation,” that is, systematically altering one factor at a time.86 For example, in one of the studies of a model biplane, the procedure involved keeping the sections, spans, chords, and the distance between the wings constant while altering the angle of stagger in order to try to isolate its effect on lift.87 But there were also bodies of important and sophisticated theoretical work waiting to be explored. The provenance of this theoretical work lay almost exclusively in the achievements of Cambridge mathemati­cal physics. Predictably, the orientation toward the fundamental, theoretical problems of aerodynamics was swept aside in 1914 by the demands of the war, which gave precedence to short-term, practical investigations. Before the cataclysm, however, in the period between 1909 and 1914, theory testing provided the focus for much of the research.

The theories in question concerned two general areas: (1) stability and control and (2) lift and drag. They therefore lay in two quite distinct areas of physics—one being grounded in rigid-body mechanics, the other in fluid dynamics. I consider them in turn, beginning, in this chapter, with the work on stability and, in the next chapter, moving to the fluid dynamics under­lying the theory of lift and drag.

To the scientist an aeroplane is merely a complex body moving through a fluid, and until he understands how a simple body moves he has no chance of understanding the fundamental principles of aeronautics.

g. i. taylor, “Scientific Method in Aeronautics" (1921)

The research agenda drawn up at the Admiralty and endorsed at the first meeting in the War Office accurately prefigured the approach that was to be adopted by the members of the Advisory Committee in their work on lift and drag. The immediate research aim was to provide a mathematical analysis that would predict the forces exerted on a flat or curved plate immersed at an angle to a flowing fluid. Of course, this was not the ultimate aim. The plate was to function as a simple model of an aircraft wing, and the mathematically idealized fluid, necessary to perform the calculations, was to act as a model of the air. To calculate the forces, researchers needed a precise and quantitative picture of the flow around the wing. What would that flow look like? For the British, the best available guess was provided by Rayleigh’s important work on discontinuous flow. Although the work was over thirty years old, and it was obvious to everyone that the analysis was highly idealized, it appeared to the Advisory Committee that here was the rational place to start. Initially, therefore, as far as lift was concerned, all the research effort of the ACA, both theoretical and experimental, went into studying the theory of discontinuity. I now describe this work and then, later in the chapter, contrast it with the ideas about lift put forward by the leading representative of the “practical men.” The contrast in style is stark.

Lanchester’s Aerodynamics was reviewed anonymously in Nature on August 18, 1908.46 The overall judgment was ungenerous and negative. No reader was likely to come away with the idea that the book contained striking insights into the nature of flight but instead that Lanchester was proposing a theory that was neither original nor successful. The theory was, perhaps, the product of a lively mind, but not a mind whose powers could be relied upon. The parts of the book that contained Lanchester’s most characteristic opinions were de­scribed by the reviewer as “the more shaky theoretical chapters” (338).

There was qualified praise for some of the more empirical sections, which described Lanchester’s experiments on viscosity and skin friction. The glider experiments, conceded the reviewer, gave results that were “remarkably con­sistent.” Lanchester’s account of the “chief methods and results of hydrody­namics,” which lay at the basis of his theory of lift, were described as “on the whole very clearly written,” but the reader was warned that Lanchester was “not, however, content to follow orthodox theory.” It was in chapter 4 of the book, noted the reviewer, that Lanchester “leaves behind the solid ground of orthodox theory” and “attempts to work out the motion of a curved lamina,” that is, a winglike surface (338). Furthermore, Lanchester’s originality was challenged: “It seems to us that the author is wrong in claiming to be the first to give a theory of the motion of curved surfaces, and [in claiming] that Lilienthal had only practical acquaintance with the curved form, for Lilien – thal clearly realised that the effect of curvature was to diminish eddy motion and to give an increased upward pressure due to the centrifugal force of the air. The theory has been worked out mathematically by Kutta, and his results are in fair agreement with Lilienthal’s experiments” (338).

The reviewer then turned to Lanchester’s own explanation of how a curved plate generates lift. It was introduced and dismissed in one sentence: “The author of the present volume attempts to work out the problem by applying the theory of cyclic motion to the motion of a surface in two dimensions, but it is difficult to see how this can have any application to the case of a lamina moving in free air” (338). Before looking into this expression of doubt I must address two preliminary points that concern the reviewer’s mention of Kutta. First, it looks as if the reviewer did not appreciate that Kutta had put forward a cyclic theory. Second, Kutta’s main contributions were published in 1910 and 1911, two or three years after the review. So what was the reviewer’s source?47

Other than personal contact, there were two possible sources of informa­tion. One was a brief account of his work that Kutta himself published in 1902 in the Illustrirte Aeronautische Mittheilungen.48 He gave his main results in the form of a complicated and opaque formula (not the simple product of density, circulation, and free-stream velocity). Kutta said that to reach the given formula he had used conformal transformations, but the assumptions behind his analysis were not explained. The other source was a footnote ref­erence to this article by Sebastian Finsterwalder, Kutta’s research supervisor at the technische Hochschule in Munich.49 Finsterwalder had contributed the article on aerodynamics to Felix Klein’s multivolume Encyklopadie der Math – ematischen Wissenschaften. The relevant volume had been published before the Nature review appeared. The cyclic character of Kutta’s theory was not apparent in the 1902 paper, though its relation to Lilienthal’s work was explic­it.50 The same holds true of the Finsterwalder reference: there was no men­tion of the role of circulation. If these were the sources used, it could account for the misleading way in which Kutta was invoked in the review.

Why did the reviewer find it “difficult to see” how an account of a two­dimensional, cyclic motion could have any application to the motion of a lamina in free air? The reasons behind the difficulty were not explained, so it is necessary to make a conjecture about the argument that was probably in the reviewer’s mind. The worry was about the move from two dimensions to three dimensions. Why should there be a problem about generalizing an ac­count of cyclic or vortex motion in this way? The answer lies in the properties of the space around the wing that mathematicians call “connectedness”—a topological theme with which all Cambridge-trained mathematicians would be familiar.51

Connectedness refers to the conditions under which a contour in the form of a closed loop can be shrunk into a point or stretched and distorted so that it coincides with another closed loop. A “simply-connected” space is one in which every closed loop can be changed into any other closed loop without going outside the space. A “multiply-connected” space is one that is divided by barriers so that it ceases to be true that any two arbitrary loops can be made to coincide. Now a loop enclosing the infinitely long wing cannot be unhooked from it. It can be transformed into any other loop that is itself already around the wing, but it cannot be transformed into a loop that does not go around the wing. The space around an infinite wing is thus “doubly connected,” while the space around a finite wing is “simply connected.”

The move from a two-dimensional analysis to a three-dimensional analy­sis thus involves a move from a multiply connected space to a simply con­nected space. But why should this matter? A mathematically sophisticated re­viewer will have known that, in a simply connected region, the only possible form of irrotational motion is acyclic.52 In an acyclic motion there is no circu­lation and hence no lift. The reviewer seems to have assumed that Lanchester was exploiting a special, topological feature of two-dimensional flow but was then illegitimately applying the analysis to the three-dimensional case.53 This assumption may explain why it was “difficult to see” how a theory of cyclic motion in a surface of two dimensions could have any application to a lamina moving in free air, that is, in three dimensions.

Was Lanchester’s work really vitiated by these considerations? The answer is no. If this was the reviewer’s argument, it was wrong. Lanchester had at­tended with some care to issues of connectivity. He stated explicitly that “we are consequently confined, in an inviscid atmosphere, strictly to the case where the aerofoil is of infinite extent, for a cyclic motion is only possible in a multiply connected region” (162).

How did Lanchester, having formulated the topological problem for himself, get round it? He needed some way to render the space of the three­dimensional case multiply connected. Lanchester did this by appeal to the trailing vortices issuing from the wingtips and reaching back to the ground. This method divided the space in such a way as to destroy its simple con – nectivity.54 In figure 81 of his book (175), Lanchester gave a clear diagram of the vortices reaching back from the wingtips to the ground. But if Lanchester had anticipated and solved this problem, there was still another issue left. If circulation now makes mathematical sense, there is still the physical problem of how it gets started. Lanchester conceded that, as long as the atmosphere was viewed as an inviscid fluid, his vortices could be neither created nor de­stroyed. Such a system, he said, “in a fluid that is truly inviscid would be un – creatable and indestructible” (174). His response was to appeal to the viscos­ity of real fluids: “In dealing with a real fluid the problem becomes modified; we are no longer under the same rigid conditions as to the connectivity of the region” (175). Lanchester’s remarks were perceptive, but the problem of the creation and destruction of vortices, and thus the problem of how circulation could arise, would continue to haunt the theory.

Lanchester came to loath Bairstow and what he called “the Cambridge School”—a group to which he had no hesitation in assigning Bairstow, de­spite the latter’s London provenance.63 Unlike the positive comments he made about the National Physical Laboratory in 1915, in later years Lanchester ex­pressed resentment at the lack of support he had received from that quarter and identified the majority of those working there as effectively belonging to the “Cambridge School.” In a memorandum written in 1936, in which he sought recognition from the Air Ministry for his contribution, Lanchester expressed himself with some bitterness: “The trouble is, or arose from the fact, that with the exception of Lord Rayleigh, the N. P.L. did not take my work seriously. . . . They fell into the error, and for this Leonard Bairstow was mainly to blame, of casting doubt on my work, I believe because my methods did not appeal to them in view of their training. They mostly belonged to the Cambridge School, whereas I was the product of the Royal College of Science (then the Normal School of Science)” (19-20).64 He recalled that, on more than one occasion, Bairstow had asserted, during meetings of the Advisory Committee for Aeronautics, that “we do not believe in your theories” (20). In an earlier letter of 1931 to Capt. J. L. Pritchard, the secretary of the Royal Aeronautical Society, Lanchester referred to “that man Bairstow who would have nothing of the vortex or cyclic theory and took every occasion when I was a member of the Advisory Committee to laugh and jeer at it.” 65

The minutes of the Advisory Committee do not contain any specific re­cord of episodes of this kind.66 Whether those writing the minutes drew a veil over such exchanges or whether Lanchester’s memory was at fault is im­possible to determine. Nevertheless there is no reason to doubt the essential accuracy of Lanchester’s account, and the minutes contain clear evidence of Bairstow’s opposition. There is also ample corroboration in the public realm. As J. L. Nayler, the secretary of the committee, put it, in his early years Bair­stow was “a dominant and almost pugilistic character.”67 In another letter to Pritchard, Lanchester left no doubt as to where he placed the blame for the opposition to his work. “The whole thing,” he asserted, “originated with Bairstow backed up by Glazebrook.”68

The personalized focus of this explanation has been taken up by others. This was the line taken by J. A. D. Ackroyd in his Lanchester Lecture of 1992. After giving an authoritative account of Lanchester’s contributions to aerody­namics, Ackroyd posed the question of why there was so little interest in the circulatory theory. “Perhaps part of the problem,” he suggested, “lay in the personalities involved.” 69 Ackroyd, however, did not place all the emphasis on Bairstow’s personality but noted the role of Lanchester’s own strong per­sonality and his inclination to be critical of Cambridge and London graduates and the work of the NPL. Perhaps, Ackroyd concluded, there was a mutual an­tipathy between the persons involved. In developing this argument, Ackroyd cited and endorsed the psychologically oriented explanation that had been advanced some years previously by the eminent applied mathematician Sir Graham Sutton FRS. Sutton pointed to what he called Lanchester’s “isolation” and put this down to Lanchester having been one of the great “individualists” of science. “Throughout his life he remained an individualist, perhaps the last and possibly the greatest lone worker that aerodynamics will ever see.”70

The clash of personalities must be part of the story, but can this really be the explanation of the opposition to the cyclic theory? I do not believe that it can. Consider the role of Bairstow’s personality. In the survey that I gave of the reasons advanced against Lanchester, it is clear that Bairstow’s arguments were aligned with those offered by others, such as Taylor, Cowley, Levy, and Lamb. Later I shall add more names to this list. I have seen no evidence that suggests they shared Bairstow’s main personality characteristic, that is, his aggressiveness. They had their own, quite different, personalities. Levy, for example, always said Cambridge was an unattractive place where the math­ematical traditions were too “pure” for his tastes. With his Jewish and Scot­tish working-class background, he said he did not feel socially or politically comfortable in Cambridge and declined the chance to do postgraduate work there. Levy’s class consciousness and bitterness at the blighted lives he had witnessed in the slums never left him.71 After graduating from Edinburgh, however, Levy used his scholarship funds to visit Gottingen (where he met von Karman) and then took himself to Oxford to work with the Cambridge – trained Love. The relation between Levy’s personal feelings and this career trajectory is not easy to fathom,72 but perhaps we do not need to understand such matters. What can be said about all these diverse and complex person­alities is that they all took a similar stance on the central, technical problems that were in question. They shared professional opinions and judgments, not individual personality traits. The explanation in terms of personality, there­fore, breaks down. The candidate cause (personality) varies, but the effect (resistance to Lanchester’s ideas) stays the same. This means that we must look elsewhere for the real cause.

What, in any case, would be the basis of an account that rested on an appeal to personality? No one believes that certain psychological types are selectively attracted to this, that, or the other preferred pattern of fluid flow, whether viscid or inviscid. Those who invoke “personality” generally do so in order to explain the disruption of a process of rational assessment that (it is assumed) would otherwise have proceeded in a different way. It is offered as a way of explaining why things went wrong. It is meant to explain why a theory was rejected when it should have been accepted, and the answer is found in individual psychological traits. But given that the assessment of Lanchester actually rested on the appeal to shared standards, common to a group of otherwise diverse individuals, this explanatory approach bypasses the most salient feature of the episode. Its outstanding characteristic was its systematic and shared nature. It had the character of a concerted action by a group.

A further point needs to be stressed. An examination of the technical ar­guments that were used against Lanchester suggests that the response to his work was not a disruption in the rational working of science but a routine ex­ample of it. It was orderly, consistent, and reasoned and drew upon a refined body of received opinion and technique. It is true that some of the complex­ity was factored out of Lanchester’s text, but that again was a consistent and shared feature of the response, not an individual variable. Personality played its part, but only by giving a different tone, and a different degree of intensity, to the expression of a central core of repeated, and overlapping, argumenta­tion. The common content of the arguments derived not from individual psychology but from participation in a shared scientific culture.

Lanchester himself hinted at an explanation of this kind. As well as his explicit and angry psychological account, focused on his irritation with Bair – stow, there was also an implicit, more sociological dimension to his account of the resistance to his theory. This aspect surfaced in his reference to the “Cambridge School” and the common background of training of the scien­tists at the NPL. We should also recall his 1917 discussion of the organizational characteristics of well-conducted aeronautical research. This, too, can be read for its bearing on the resistance encountered by Lanchester’s work. His central preoccupation was that the different parties to the process of research should confine themselves to their proper spheres of competence. No good would come, he argued, of mathematicians and physicists encroaching on territory outside the (narrow) limits of their expertise. What could have been in Lanchester’s mind? What examples of invasive physicists might he have cited? The public confrontation with Bairstow, two years previously, when they clashed over the proper scope of a theory of lift could not have been far beneath the surface. Was it necessary to find a universal law of nature, as Bair – stow wanted, or would a specialized, practically oriented approach suffice, as Lanchester believed? Whether or not this was the example in Lanchester’s mind, it illustrates the general problem to which he was referring, namely, the problem of the division of labor.

The division of labor generates a diversity of specialized perspectives and localized forms of knowledge. Professional subgroups and disciplinary divi­sions such as those between mathematical physics and technical mechanics are instances of this general phenomenon. What happens when the product of one of these subgroups and perspectives is assessed from the standpoint of another, different subgroup with a different perspective? We have here all the preconditions for a small-scale culture clash. Has the knowledge claim been properly understood, or has it been misinterpreted? Is a contribution to one project being assessed (deliberately or unwittingly) by criteria more appro­priate to another project? If I am right, this is exactly what happened when Lanchester’s work was assessed so negatively by the “Cambridge School,” and it was this problem (although it was not the only problem) that Lanchester was addressing when he discussed the proper organization of aerodynamic research.