Category The Enigma of. the Aerofoil

Kutta now carried out the procedures for which he had prepared the ground. He began on the z-plane and specified the detailed geometry of the wing. It was to be an arc of a circle of radius r subtending an angle of 2a. This gave the coordinates of the endpoints A (the leading edge) and B (the trailing edge). The straight-line distance between A and B was the “chord,” and the highest point of the arc was to be 1/12 of the chord. Kutta chose to place this high­est point at the origin of the coordinate system. He then began the process of transformation. First he used a transformation in which every point was replaced by its reciprocal. Points on the z-plane were linked to those on the z’-plane by the formula z’= 1/z. This had the effect of turning the finite, cir­cular arc into what appeared to be two straight lines. One of them ran parallel to the positive part of the x-axis while the other ran parallel to the negative part of the x-axis. Both were at the same height above the axis. They started at equal distances from the y-axis (that is, there is a gap in the middle), and the lines went off to infinity in opposite directions.

It would have helped the reader of Kutta’s paper if, at this point, he had provided a diagram. Given the pedagogic values of the technische Hoch – schulen, he would surely have drawn pictures of such transformations on the blackboard when he presented them in lectures. Most mathematicians reading such a paper would sketch the appropriate figures, at least until the transformation had become routine for them. To help us follow Kutta’s argu­ment, I exploit an example of this practice. Sometime in the 1920s a young Cambridge mathematics graduate named Muriel Barker had occasion to work through Kutta’s article. She carefully wrote out the reasoning, some-

times filling in the steps needed to get from one line to another. She also sketched the conformal transformations. These handwritten notes have sur­vived, and one page from them, containing the sketches, is reproduced here as figure 6.2. Muriel Barker will appear again, later in the story, when the reasons for her interest become apparent. For the moment her notes can help us follow Kutta’s thought processes.

On the top left of the page of the notes is a figure labeled z-plane. It is a drawing of the Kutta-Lilienthal wing with the leading edge labeled A and

the trailing edge labeled B. The effect of the transformation z’ = i/z is shown next to it in the diagram, on the top right of the notes, labeled z’-plane. No­tice how the arc has become two straight lines and the leading and trailing edges A and B of the wing have become the endpoints A’ and B’ of the lines. Following his overall plan, Kutta next mapped these lines onto the t-plane where it would eventually link up with the transformed circle. This he did by using the Schwarz-Christoffel transformation. I have described how this transformation played an important role in the mathematical development of the theory of discontinuous flow. It was central to Greenhill’s massive report on this theory for the Advisory Committee for Aeronautics. Kutta used the transformation in a different way and in the service of the circulation theory. He needed it to construct the central arch of his mathematical bridge. The formula of the transformation can be seen about halfway down the page of notes in figure 6.2. It takes the form

The letter C is a constant, and a and b correspond to the endpoints of the wing. Immediately to the right of the formula is a sketch of the result of the transformation produced by applying this formula. The lines on the z’-plane have become the axis of the t-plane. The new line is shown as dotted in the figure, and the points corresponding to A’ and B’ have been marked in. All that was needed now was to work from the other end in order to map the circle onto the t-plane. The inferential bridge would then have been con­structed according to plan. The circle in the Z-plane is drawn on the bottom right-hand corner of the notes. The formula

Z+1

Z-1 is the transformation linking Z and t. This can be seen in the notes standing to the left of the drawing of the circle. Kutta’s aim might be described as getting from the figure at the bottom right to the figure at the top left of the page, but because he could see no way of doing this directly, he made the transition indirectly, by means of the other figures.

Coming back from the Barker notes to the original paper, we see that Kutta was now in a position to evaluate the constants in his formula in terms of the assumed velocity and direction of the free stream relative to the wing. He could also arrive at a value for the circulation on the assumption that the trailing edge is a stagnation point, that is, that the flow does not have to curl around the rear edge. This gave him the following expression for the
all-important circulation, which, in the notation used by Kutta, is 2ПС. The formula came out as

Circulation = 4nVr sin—sin I —+B,

2 ^ 2 H)

where V is the velocity, a the half angle of the arc that constitutes the wing, and в the angle of incidence of the wing to the free stream. The circulation is thus calculable from known or knowable quantities.

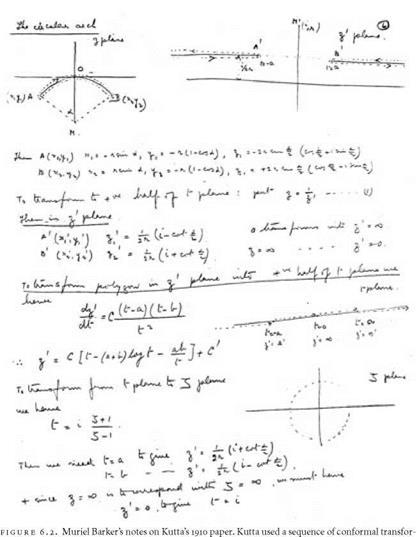
The law of Biot and Savart received a number of further aerodynamic appli­cations before the outbreak of World War I. All of these were published in the Zeitschrift fur Flugtechnik and came from the Gottingen group. Four of them were by Albert Betz and one by Carl Wieselsberger. I describe them briefly, keeping to the chronological order of their appearance.

In September 1912 Betz published some wind-channel results that showed that a wing operating in the vicinity of the ground would experience an in­crease in lift.61 Betz showed this by testing a model wing in a channel fit­ted with a false floor that could be raised or lowered. The phenomenon was an important one. Aircraft necessarily fly near the ground on landing and takeoff. Pilots were aware that there was a change in flying characteristics produced by these circumstances, but the nature of the change was little understood. This “ground effect” explains why an overloaded aircraft can sometimes take off with apparent success and then fail to gain height, with disastrous consequences. It also explains why some early aircraft could “fly” but never got more than a few feet above the ground.62 Betz also wanted to get a quantitative estimate of the effect of the walls of a wind channel on the measurements that were carried out in the course of experimentation. He showed that Prandtl’s new theory could lead to rough but quantitative pre­dictions that were confirmed by experiment. (The results were approximate, Betz suggested [220], because the “horseshoe” model ignored the downward motion of the trailing vortices.) Both of the subjects that Betz broached in his brief paper were to become a matter of enduring concern and research in subsequent years.

In January 1913, Betz published a second study, this time of the lift and resistance of a biplane.63 Whereas Foppl had used the Biot-Savart law to study the effect of the induced velocity on the tail wing, Betz now used the same approach to study the mutual interaction of wings that were positioned one above the other. The central point about the application of Prandtl’s approach to a biplane is that the trailing vortices from the upper wing will generate an induced resistance not only in the upper wing itself but also in the lower wing, while the trailing vortices from the lower wing will likewise affect both wings. Furthermore, if the wings are not located directly one above the other, the bound vortex corresponding to the wing itself (and not just the trailing vortices) will have to be taken into account when computing the induced velocity and induced drag on the other wing.

With the exception of Kutta’s second, 1911 paper, this work represented the first serious engagement with the theoretical aerodynamics of the biplane and the difficult problem of the mutual interaction of the different parts of an aircraft. It will be recalled that the “practical men” in Britain stressed holistic effects to justify their conviction that only the intuition of the engineer could cope with the problems of airplane design. Scientists and mathematicians, they said, simplified problems by studying one part at a time, which doomed them to failure. Such a procedure ignored the all-important effects of inter­action. Perhaps (had they known about it) the “practical men” would have been impressed to be told of the progress that was being made in Gottingen. Here engineers, such as Betz, were using the Biot-Savart law to put the study of interaction on a mathematical as well as an experimental basis.

Betz carried out wind-channel measurements of the lift and resistance of a set of two wings rigidly fastened into a biplane configuration. He studied (a) the effect of varying the distance apart of the wings, (b) the effect of giv­ing the wings different angles of incidence from one another (decalage), and (c) the effect of placing one wing ahead of the other (stagger). He found that the effects were small within the range he studied, though the most signifi­cant variable was the stagger of the wings. One of his practical concerns was to form some idea of the relative merits of monoplanes and biplanes. He summed up his results in four propositions: (1) A biplane arrangement with wings of equal span always has a less favorable ratio of lift to resistance than one of the wings taken separately. (2) A biplane can have advantages over a monoplane when the rest of the resistance of the aircraft, for example, a bulky fuselage, is taken into account. (3) A biplane is at an advantage if a high lift at low speeds is required. (4) The greatest maximum lift is obtained when

the upper wing of a biplane is placed ahead of the lower wing and is given a slightly smaller angle of incidence than the lower wing. All of these results, said Betz, were rendered intelligible by Prandtl’s theory, and the empirical graphs of lift and resistance were duly accompanied by theoretical curves calculated from the theory.64

In neither of his papers did Betz specifically mention, or illustrate the use of, the Biot-Savart law. He alluded to the horseshoe model but revealed none of the mathematics involved in his calculations. Like Foppl he prom­ised the reader that a fuller account was to follow from the pen of Prandtl himself. The Great War began in July 1914, but there seemed no immediate concern with secrecy. In a paper that appeared in August 1914, Wieselsberger preempted Prandtl and stated the Biot-Savart law explicitly and illustrated its application.65 He asked why birds often fly in a V formation. He did not man­age to answer the question, but he did succeed in laying out the basic ideas, and the basic mathematics, of Prandtl’s theory. In approaching the problem of formation flying, Wieselsberger ignored the beating wing motion involved in bird flight and treated birds as small airplanes. He then followed Prandtl and treated the airplane as a horseshoe vortex. By the use of the Biot-Savart law he showed that on either side of the horseshoe vortex there would be an updraft. This, he argued, allowed another wing, positioned to one side of the first wing, to operate at a more favorable angle of attack. This lowered the component of induced resistance in the direction of flight. On the basis of some plausible numerical assumptions, he made a quantitative estimate of the advantages to be derived from flying in the updraft of neighboring birds. His overall model, however, led to the conclusion that side-by-side flight would be just as efficient as the V formation.

In September 1914 Betz produced a study of wings with a sweepback and a twist at their ends,66 a configuration frequently used by designers of German aircraft at that time. The name Taube, or “dove,” was given to such machines. In Betz’s paper there was a passing reference to yet another formula attrib­uted to Prandtl and his new theory, though again no derivation was given. The formula concerned the minimum glide-angle that could be expected for a wing of given span and lift. The main result of Betz’s experiments on a range of Taube-style wings was to confirm the near optimum character of very sim­ple, rectangular wings. Having neither twist nor sweepback, such wings also had an economic and practical advantage: they were easy to construct. The glide coefficient (given by the ratio of resistance over lift) was not signifi­cantly improved by sweepback or twist, though Betz did find they improved longitudinal stability.

Perhaps because the promised theoretical paper from Prandtl was not forthcoming, Betz finally published his own account of the mathematics underlying his papers. Titled “Die gegenseitige Beeinflussung zweier Trag – flachen” (The mutual influence of two wings),67 the work appeared in the Zeitschrift fur Flugtechnik for October 1914. Betz concentrated on the case of the staggered biplane with wings of equal span where the upper wing was positioned ahead of the lower wing. Because the analysis proceeded on the assumption that each wing and vortex system could be represented by the simple “horseshoe” schema, the only real novelty in the paper lay in the more complex geometry of the computations, but the explicit development of the mathematics of the theory demonstrated its applicability to what was then a vitally important form of aircraft. It was clear that Prandtl and his colleagues now had a theory that could be used to predict the induced resistance of bi­planes, or triplanes, using only the wind-channel data for a single wing.

‘In the same year, 1914, Wieselsberger also published a survey article that described the state of knowledge in German aerodynamics with respect to lift and drag. It did not appear in the ZFM but in an Austrian journal, the Osterreichische Flug-zeitschrift.68 The article covered both two-dimensional and three-dimensional theory and took the reader through the work of Kutta, Joukowsky, Deimler, and Blumenthal and up to Prandtl’s horseshoe vortex. Wieselsberger’s survey effectively brought up to date an earlier survey by Reissner, of the TH in Aachen, which had laid stress on questions of stabil­ity and propeller theory.69

The international situation had been deteriorating throughout 1914, and British statesmen, such as Lord Haldane, became increasingly worried about the “war party” surrounding the German kaiser.70 With the threat of war, it was ever more important for European countries to monitor the technol­ogy of their potential enemies. If anyone had wanted to keep an eye on Ger­man aviation, the papers of Foppl, Betz, and Wieselsberger would have given them all they needed to know about the general state of scientific knowl­edge in the field of aerodynamics. These publications would have made clear that the circulation theory of lift was wholly taken for granted in Gottingen and the German-speaking world. Collectively, the publications showed that the theory had been developed to the point where it was being applied to problems of practical importance. Betz’s theoretical analysis of the biplane, however, was the last of the Gottingen research papers to appear in an open and accessible format. Thereafter they would be hidden away from public view in the Technische Berichte, published in individually numbered copies by the military authorities and marked Geheim—“secret.” In the meantime, the Gottingen results were in the public realm and were available to anyone in Cambridge or London who cared to study them.

Making the Horseshoe Model More Realistic

Prandtl never produced the promised article in the Zeitschrift fur Flugtech – nik. This was not because he harbored reservations about the approach. On the contrary, he was happy to produce accounts for general surveys, for ex­ample, in volume 4 of the Handworterbuch der Naturwissenschaften published in 1913. The handbook was an encyclopedic survey of the state of the natural sciences and contained articles by both Fuhrmann and Prandtl. Fuhrmann wrote on hydrostatics, and Prandtl wrote on fluid dynamics.71 In his contri­bution Prandtl gave an explicit account of the circulation theory and pre­sented a graph contrasting Kutta flow with Kirchhoff-Rayleigh flow (136). He also cited Lanchester’s work and gave a diagram (112) that laid out the qualita­tive basis of the horseshoe model, though the Biot-Savart law was not men­tioned by name. Why the hesitation? The simple horseshoe model was clearly in a provisional state and was still undergoing revision. It contained formal features that compromised both its empirical adequacy and its practical util­ity. Despite the successes of the theory, it would have been understandable if Prandtl had wanted to remove these limitations before presenting the ap­proach to a specialist readership. The time was hardly ripe for an authorita­tive presentation, which may explain the non-appearance of the article. Then the war intervened, and the form and level of presentation at which he seems to have been aiming were not achieved until 1918.

The problems with the “horseshoe” vortex were both mathematical and physical and were closely interconnected. Mathematically there was the dif­ficulty arising from the singularity in the Biot-Savart formula which has al­ready been remarked on, that is, the problem that arises when h = 0. The formula implied that the velocity of the downwash at the wingtips became infinite. The formula yields this result because of the uniformity of the vortex distribution implied by the model, that is, the constant value of the circula­tion along the bound vortex and hence along the span of the wing. This was a physically false picture. The existence of lift implies that there must be a greater pressure beneath the wing than above it, but the finite length of a real wing allows the air at high pressure beneath the wing to move round the tip to occupy the lower-pressure region above the wing. Such freedom of movement ensures that the pressure difference between the upper and lower surface will be zero at the tips. There will therefore be no lift at the tips and hence no circulation. Circulation cannot be constant along the span in the way that was assumed in the simple horseshoe model; it must fade away to zero at the tips.

Prandtl’s problem was to find a model with a more realistic lift distribu­tion along the span of the wing. His response was ingenious. He complicated the simple horseshoe model by introducing a number of horseshoe vortices laid out in the fashion indicated in figure 7.11. (A similar figure was used in an early article by Betz.)72 Starting from a single “horseshoe” whose span co­incided with the full span of the wing, he added others of smaller span. The parts of the vortex that lie along the span are to be thought of as piled on top of one another. In this way the constant distribution of circulation along the span is replaced by a variable, stepwise distribution with a maximum at the midpoint. The arrangement had the consequence that vortices now trailed from a number of points along the rear edge of the wing, rather than merely at the wingtips. This stepwise model, however, was only the starting point of Prandtl’s line of reasoning.

Prandtl did not simply introduce a number of horseshoe vortices such as the five in the diagram, or even 50 or 500. He introduced an infinite num­ber. He postulated an infinite number of vortices of infinitesimal strength. The vortices were infinitesimal for two reasons. First, an infinite number of vortices of finite strength would result in the absurdity of a wing with infinite circulation and infinite lift. Second, he needed the circulation and the lift at the tips to approach zero. A stepwise model with finite vortices would merely reproduce the problem that dogged the original. The vortices had to become infinitely small at the wingtips. Along the span of the wing the infinitesimal vortices were assumed to be compressed into a single line of bound vortic – ity (of varying strength) called the lifting line. These refinements made it possible to imagine a smooth, rather than stepwise, lift distribution that was amenable to mathematical treatment. To accord with the known facts, the

smooth lift distribution had to have a maximum lift at the midpoint of the span and approach zero lift at the wingtips.

Having described Prandtl’s refined model in qualitative terms, I now show how he expressed these ideas in mathematical terms. This account will pre­pare the ground for the next two sections, which describe the technical and mathematical heart of the Gottingen achievement.

Suppose the wing has a span b and lies along the x-axis of a coordinate system so that it runs from x = —b/2 to x = +b/2. The distribution of the circu­lation can then be represented by Г(х). The symbol indicates that, for every value of x along the axis between the wingtips, there corresponds a specific value of Г, the circulation. Thus Г(о) is the value at x = 0, the origin, which, following convention, is taken as the center of the wingspan. It is known from experiment that the lift is at its maximum value at this central position. Because it plays an important role, it is customary to give the circulation at this point a special designation and write Г(о) = Г0. The lift and hence the circulation is zero at the tips, so that Г(—b/2) = 0 and Г(+Ь/2) = о. For the moment, and for the purpose of conveying the main outlines of Prandtl’s theory, the actual shape of the lift distribution need not be given in more detail than this. The mathematical shape described by the function Г(х) will, for the moment, remain unspecified, but it will be some smoothed-out ver­sion of the shape made by the stepwise lift distribution. The details are re­served for the next section. For the remainder of this section, the distribution is simply referred to as Г(х) so that the general structure of the mathematical reasoning can be rehearsed. My aim is to show, in general terms, how Prandtl used the Biot-Savart law to calculate the lift, the induced velocity, and the induced drag.

The first step was to relate each of the infinitesimal horseshoe vortices to the Biot-Savart law. The relevant version of the formula for a vortex of finite strength has already been stated, namely, w = —r/(4nh). Because the analysis was now to be applied to infinitesimal vortices, the formula became dw = —dr/(4nh). The goal was to calculate the downwash at some specified point on the wing with the coordinate, say, x = x’. All of the infinite number of trailing vortices (each coming away from the wing at some point with its own specific x-coordinate) will contribute to the downwash at the point x’. The Biot-Savart law gave the (infinitesimal) contribution dw made by each of these infinitesimal vortices. The perpendicular distance h in the formula needed to be re-expressed as (x’ — x). This was the distance between the point on the wing from which the infinitesimal vortex emerges and the point x’ at which the downwash was to be found. A process of integration that adds the contribution of all the infinitesimal trailing vortices would then give the total

downwash at X. A further calculation, and a further integration, was needed to get the downwash for the entire wing, that is, for all the points like x’ which lie along the span between x = – b/2 and x = +b/2.

The procedure that has just been sketched was based on the assumption that the quantity dr used in the Biot-Savart formula corresponded to the strength of the infinitesimal vortex at the arbitrary point x. How was this infinitesimal strength to be expressed? The answer was that the strength of the element of trailing vorticity issuing from a point x was equal to the change of vorticity on the wing at that point. This can be explained by going back to the stepwise model of a finite number of finite vortices that was shown in figure 7.11. First the outer horseshoe is put in place. Suppose this has strength Tj. Then the second horseshoe is added, which has strength Г2 and a slightly shorter span, then Г3 is added, which again has a slightly shorter span, and so on. Consider the two points on either side of the origin of the x-axis from which the trailing vortices of strength Г2 emerge. These are the points at which the distribution of circulation changes by an increase of the amount Г2. Thus the strength of vorticity trailing from the wing at that point equals the change in vorticity around the wing at that point.

This “strength equals change” rule holds even when there are an infinite number of infinitesimal horseshoe vortices. The distribution of circulation along the span is given by the curve r(x), so the change in circulation is the slope of the graph of r(x) multiplied by the distance over which the slope reaches. The slope is дГ/dx, and the distance is dx, so the change whose value is sought is dr = (дГ/dx) dx. This expression gave the strength of the cir­culation or vorticity to be entered into the formula for the Biot-Savart law. The infinitesimal contribution of the vorticity at x to the downwash at x’ was therefore

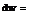(ЭГ / dx ^jdx 4n(x’ — x)

The total downwash at the point x’, designated by w(x’), is the integral of all of these infinitesimal contributions, summed over all the vortices issuing from the whole span of the wing. Thus,

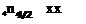/X 1 +if2(dr / dx )dx w(x ) = -— I Л-Ж J

The above integral has a singularity at x = x’, when the denominator becomes zero, but the integration could be carried out in such a way as to avoid this problematic point.

Given the downwash it was then possible to calculate the induced angle of incidence at X. This angle, ф, follows from the value for w(x’) because it was simply the angle made by combining the downward induced velocity with the free-stream velocity. The ratio of the two speeds gave the tangent of the angle ф, but because the angle was small, the angle and tangent could be equated. The induced angle of incidence was

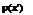w(x’) V ‘

The lift distribution could now be related to the overall lift and induced drag. Recall that for an infinite wing the flow at every cross section resembles that at every other cross section. The lift per unit length is constant and is given by the Kutta-Joukowsky formula as L = рГV. Prandtl took this formula to apply to each separate, infinitesimal element of a three-dimensional wing, with the proviso that the circulation would vary from element to element according to the distribution Г(х). The overall lift could then be represented by the integral of all the elementary lifts: dL(x) = р V r(x)dx. Thus,

+b/2

Lift = pV J Г(х)dx.

—b/2

Each point on the wing would generate an element of downward velocity and would thus be subject to a slight downward slope in the local flow. The ele­ment of lift dL(x) at that point would be tilted backward (relative to the main flow) so that the resultant force possesses a component opposing the motion. This was the induced drag. The induced drag at a given point x depended on the induced angle of incidence ф at that point. The component of induced drag resulting from the backward tilt equals dL(x) sinф(x). For small angles the sine of ф is equal to ф itself, so the element of induced drag was dL(x) ф^). Thus the total induced drag was given by the integral

+ b/2

Drag = pV J Г(х)p(x)dx.

— b/2

This relation could be expressed in terms of a coefficient of induced drag by dividing the value of the drag force itself by Уг р V2F, where F is the area of the wing. This gave the coefficient of induced drag as

2 +b/2

CD = — J T(x)<p(x)dx.

—b/2

It will be evident from these formulas that a closely knit structure of theoretical relations was emerging in Gottingen which connected lift, drag, span, and the distribution of circulation along the span of a wing. For the purposes of exposition I have only presented this structure in a schematic form. The mathematical formulas just given all depend on the distribution of the circulation, Г(х), but the actual character of the function governing the distribution has remained unspecified. All that the above formulas entail is that if the distribution Г(х) is given, then the lift, the induced angle of incidence, and the induced drag can be calculated. Only when the distribu­tion is specified will the theory will have real content. The next question is: How was the distribution of lift and circulation found? How is Г(х) to be defined?

Prandtl was not vastly outstanding in any one field, but he was eminent in so many fields. He understood mathematics better than many mathematicians do.

max munk, “My Early Aerodynamic Research” (1981)1

After Glauert and McKinnon Wood had presented the reports on their Got­tingen visit, discussions continued in the Aeronautical Research Committee as the British experts sought to mobilize a collective response to the Ger­man wartime achievements. These (sometimes sharp) exchanges took place in the monthly meetings of the committee and its subcommittees that were held in London. The Cambridge contingent made the journey to London together by train and engaged in lively aeronautical debate en route. “I fear we must have been a pest to our fellow travellers,” recalled one.2 The upshot of the committee meetings are recorded not only in the minutes of their dis­cussions but also in the confidential technical reports circulated among the participants. The content of the technical reports sometimes surfaced in the published Reports and Memoranda issued by the committee and sometimes, in the case of especially important results, in leading scientific journals. A number of the main experiments done in this period appeared in the Philo­sophical Transactions of the Royal Society and in the Proceedings of the Royal Society. There were some significant and perplexing changes in the analysis of the experimental material as the data made the journey from the private to the public realm.

I have described how Taylor, in his 1914 Adams Prize essay, had dismissed Lanchester’s idea that the flow of air over a wing was describable in terms of a perfect fluid in irrotational motion with circulation. If Prandtl was right, then Taylor had been wrong. Led by Glauert, the postwar argument in the Aero­nautical Research Committee seemed to be going in Prandtl’s direction. The circulation theory was gaining ground. By 1923 Glauert felt able to write to Prandtl to tell him that his “aerofoil theory has certainly aroused much inter­est here and it would not be an exaggeration to say that it has revolutionised

many of our ideas.”3 But Taylor (see fig. 9.1) was not to be easily convinced that his earlier reservations had been misplaced. In the postwar discussions, he made it his job to scrutinize Glauert’s reasoning and to oppose it whenever he detected a logical gap or a questionable premise.

In paragraphs 185-99 of Philosophical Investigations, Wittgenstein imagined a pupil who is being taught to follow a simple rule, namely, the rule of “add 2.” The pupil must try to follow the rule and generate the rule-bound sequence of 2, 4, 6, 8, etc., by adding 2 to the previous member of the sequence. The pupil is taught by a familiar mixture of examples and explanations and is then encouraged to go on to produce further numbers in the sequence. Witt­genstein then imagined the pupil deviating from the expectations of the teacher and the other competent rule followers in the surrounding culture. On reaching 2,000, the pupil does not say 2,002, but 2,004, 2,008, etc. Witt­genstein studied the likely reactions of the competent rule followers and the resources at their disposal as they tried to get the deviant pupil to understand what the rule means and what the rule requires. It rapidly emerges from the analysis that just as the original rule might be misunderstood, so too might any further explanation. Such explanations also depend on a small number of illustrative examples that the pupil must use as a pattern to generate the new instances that are required. Thus, the injunction to go on in the same way merely pushes the problem back to that of defining “same.” Any attempt to furnish an algorithm for producing the next member of the sequence merely provides rules for following rules and the original problem of furnishing an adequate analysis repeats itself.10

Ultimately any attempt to furnish reasons, or to ensure that the pupil’s behavior is guided by what the rule requires, depends on the pupil react­ing normally and automatically to the training provided by those already ac­knowledged as competent. There is nothing else available, and all appeals to “reason,” “logic,” “meaning,” “implication” (as well as the concepts of “must” and “have to”) come down, in the end, to this. The basis of the pro­cess is participation in a shared practice. This applies not just to the pupil now learning the rule but to all who have ever learned it and all who now teach others and identify and correct their errors. The meaning of the rule and the compulsion of the rule depend on shared dispositions to react and shared dispositions to interact with one another in ways that ensure that in­dividual responses stay accountable and aligned. In other words, rules are conventions. To obey a rule, said Wittgenstein, is to follow a “custom” and to participate in an “institution.”

Wittgenstein did not mean this statement as a criticism of rule follow­ing or the practice of citing rules. The claim is not that rules are unreal or that rule following is a sham. He was not saying that rule followers have no reasons for what they do or, in reality, are acting for other reasons. His point was that the institution of rule following is a social reality, and his aim was to expose that reality to view. The institution is vital for, and constitutive of, cognitive and social order. Citing the rule as an explanation of why pupils act as they do, or why they should act thus and so, is the currency with which one rational, social agent interacts with another rational, social agent. The rules and reasons are actor’s categories. Invoking rules is not an idiom for describ­ing the interaction in causal terms; it is a means of acting causally within the situation. What Wittgenstein’s argument shows, and was meant to show, was that the actor’s account will not suffice if it is taken out of context and treated as if it were self-sufficient, that is, as an analyst’s account. The analyst needs to understand an interaction in terms of causes. This is why Wittgenstein ad­opted the sociological perspective. In his example he focused attention on the process of socialization. More generally he adopted the explanatory stance by invoking the concepts of convention, custom, and institution.11

Wittgenstein’s genius lay in identifying a simple example that epitomizes all the central points concerning the relation of reasons and causes. Rule following is the perfect example of rational compulsion, and Wittgenstein’s analysis can be related directly to the questions posed by critics of the Strong Program. Here, if anywhere, the case could be made for the operation of ra­tional rather than social causes. Doesn’t the rule provide a sufficient reason for the behavior of a competent rule follower? Can’t the rule better explain the behavior of the rule follower than any social causes can? It seems to give the critics everything they want. But, as Wittgenstein’s analysis shows, the critic’s case collapses into a regress of rules for following rules. For the purposes of analysis and explanation, the regress must be stopped and so the phenom­enon must be seen as a combination of psychological and sociological causes. The stated reasons and the appeal to reasons prove insufficient to explain what happens, and, for the scientific analyst, the actor’s account needs to be reconfigured in terms that acknowledge an all-pervasive and self-sufficient sociopsychological causation.

Despite the central role played by Wittgenstein’s argument in setting up the Strong Program, its critics insist on misreading the sociological approach as the claim that reason giving is spurious. Such a misreading is question begging. It amounts to making the assumption from the outset that “real” reasons and “good” reasons are not to be analyzed in terms of social causes. The categories of the “rational” and the “social” are set in opposition to one another. The critics cannot believe that rationality can be a sociological phe­nomenon in anything other than a trivial sense. They therefore counterpose the rational and the social and read the symmetry requirement of the Strong Program as an a priori exclusion of the power and presence of real reasons. From the critic’s standpoint it then seems easy to refute the sociological ap­proach. All that is needed is an example of an action authentically based on good reasons, for example, a case of rule following or a well-founded sci­entific inference. This, critics assume, refutes the preposterous generaliza­tion put forward by sociologists of knowledge. That the sociologist, following Wittgenstein, is actually challenging the dualistic assumptions upon which the critic’s argument rests is never considered.12

Although Wittgenstein’s example deals with a particular case, the lesson that can be drawn is wholly general. The lesson is that the familiar distinc­tion between “cognitive factors” and “social factors” is wrong. The injunc­tion to “disentangle” these two things is incoherent. The social factor cannot be considered “external” to the cognitive process; it is constitutive, and you cannot “disentangle” that which is constitutive. The cognitive factor in Witt­genstein’s example is, of course, the rule itself and the orientation to the rule. But what, when properly analyzed, is “the rule”? Is it something that mys­teriously exists “in advance” of the acts of following, like a rail stretching to infinity? No, said Wittgenstein, that is just a mythical picture. We must think in a different way. A rule is something that exists solely in virtue of the social practice of following the rule (just as, in economics, a currency exists solely in virtue of the practice of using the currency). The meaning and implica­tions of the rule only exist through being invoked by the actors to correct, challenge, justify, and explain the rule to one another in the course of their interactions. This is what Wittgenstein meant by calling a rule an “institu­tion.” The implication (though these are not Wittgenstein’s words) is that the rule, that is, the cognitive factor, is actually itself a social factor. Those who appeal to a combination of cognitive factors and social factors, as if they are two, qualitatively different kinds of things, are not being prudent; they are being muddled or metaphysical.13

The processes that Wittgenstein brilliantly distilled into his example are the same ones that occurred on a larger scale in my case study. That which is recognizably social, for example, the disciplinary identities, the institutional locations, the cultural traditions, the schools of thought, are not “external” to the reasoning processes that I have studied but are integral to them. They are constitutive of the step-by-step judgments by which the different bodies of knowledge were built up. Experts gave reasons to explain and justify their views and found that sometimes they were accepted and sometimes rejected. Facts and reasons that inclined the members of one group to orient in one direction inclined the members of the other group to orient in a different direction. As one would expect from Wittgenstein’s example, these accep­tances, rejections, indications, and orientations fell into patterns. The pat­terns form the customs, conventions, institutions and subcultures described in my story.14