System

Exhibit 2.12 takes us to the navigation system and to an example of another strategy for coordinating object positions: discursive (and, as we shall shortly see, pictorial) monitoring and self-correction.

How does this work? The strategy coordinates object positions in a way that suggests monitoring and self-correction will put each other right, indeed perhaps rebuild one another, should the links between them or the positions concerned start to weaken. Such is the point of the term “correction” in exhibit 2.12 and the rationale for talking of a ‘‘weapons system’’ in exhibits 2.2 and 2.13.

These terms and this strategy are both less hierarchical than those built in the table of contents. On the other hand, they echo a substan­tial literature on large technical systems in technoscience studies, a literature that lays stress on the interconnected and interlocking char­acter of technical innovation.9 The argument is that large technical systems—and here TSR2 is being treated as an exemplar-look at and talk to themselves reflexively.10 That is, they bring themselves into being and sustain themselves because they build, or take the form of, feedback systems.

Exhibit 2.13 generates components—let’s say object positions— that are coordinated to perform and stabilize the ‘‘complete TSR2 weapons system.’’ ‘‘In-built test facilities, pre-checked packages for armament, etc.,’’ such are specific objects that work together in this discursive strategy to secure the stability and continuity of the TSR2 object through a series of different positions.

The strategy takes different forms in different places. For instance, exhibit 2.13 works by colonizing alternatives, by simply obliterating them, or by rendering them irrelevant. This is the point of the talk

EXHIBIT 2.12 ”Fixing consists of comparing the computed position of the fix point with the actual positi on of the poi nt as shown by radar. Both these positi ons are shown on the navigator’s radar display and his action in comparing these pro­duces a signal proportional to the displacement between them. This signal is fed to the digital computer where it is used to correct the computed dead reckoning position and may be used to feed an azimuth correction to the inertia platform if necessary.” (British Aircraft Corporation 1962, 26)

EXHIBIT 2.13 ”The complete T. S.R.2 weapons system is designed for mobility and flexi bi lity i n operation, reversi ng previous trends towards reliance on major base facilities. It can be deployed rapidly throughout the world with nominal support and is then ready for immediate operational use to an extent depending on the level of support. In-built test facilities, pre-checked packages for armament, etc., and an auxiliary power plant for operating aircraft electrics, cooling and other systems, are used during the turn round, thereby avoiding reliance upon complex support equipment. All support equipment is air-transportable.” (British Aircraft Corporation 1962, 5)

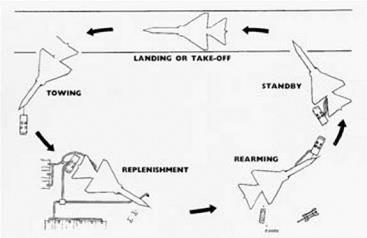
The looplike and self-sustaining character of this strategy of co­ordination is visible in exhibit 2.14. This (or so the caption tells us) is to be understood as a diagram of ‘‘turn round at dispersed or primitive airstrips.’’ In this depiction the loop starts (and ends) with landing and take-off, moves through icons that depict towing, replen­ishment, rearming, and standby, using (as the text observes) “self – contained facilities [which] can be used for normal operations.” Many coordinating conventions are being deployed here, textual and icono – graphic. But in the present context it is the arrows that are most sig­nificant. The conventions for reading these generate a viewer who is not naive but understands that the five icons for the aircraft stand not for five different aircraft but rather for one: a singular aircraft that is being displaced through time, and perhaps (though this is less clear) through space. A singular aircraft is being made that will be returned to the sky even though it is far removed from major base facilities.

A version of the same systems singularity is also deployed in one of the maps discussed earlier—the ferry-range map of exhibit 2.6. We have discussed the cartographic practices mobilized here, and also the conventions for tracing the movement of small objects onto mapped surfaces. But the latter with its lines and arrows also per­forms a further version of systems coordination. As in exhibit 2.14, this takes the form of lines and arrows which go round, in loops. The particular rhetoric of TSR2 singularity here thus not only performs an aircraft that can fly long distances once it is fueled up, but also per­forms a TSR2 that can go out, for instance from the UK, but that can also come back. This, it should be added, is not the trivial matter that it might seem, given prevailing headwinds and the need to plan for adverse conditions when seeking to land on tiny islands in the middle of the ocean.

Each of the exhibits I’ve touched on in this section contains loops. Each performs loops. And the strategy for coordination depends on the successful manufacture of loops. For, in a systems world, the world of cybernetic self-maintenance, properly built loops are re­assuring. They correct themselves. They secure an environment in which coherences may sustain themselves and that does not distort what is passed round the loop. So it is that such loops, or the connec – 28 Objects tions that afford such loops, generate what Bruno Latour (1987) calls

immutable mobiles. Objects remain ‘‘the same’’ even as they move and displace themselves.