The mid-course correction
The purpose of determining the state vector was to see if their course to the Moon was true. If it w’as not, they could do something about it. With this in mind, seven occasions were set aside in the flight plan for possible trajectory corrections; four on the way out and three for the return trip.
Having determined the state vector and calculated the current trajectory, FIDO the controller primarily concerned with planning the spacecraft’s trajectory – then brought the RTCC computers to bear on the task of working out the magnitude and direction of a mid-course correction burn, a manoeuvre that would restore their current path to the ideal. If the mid-course correction was small, as it usually was, it could be made using the RCS thrusters on the side of the service module; otherwise the SPS engine was used. On some occasions, the flight controllers deliberately started the crew’ on a trajectory that w’as slightly off the ideal in order to allow’ them to correct it using the SPS. thereby providing an opportunity to exercise the big engine and see how well it worked. Everyone knew how’ crucial this engine was, and even a short burn lasting a second or tw’o little more than a burp generated reams of engineering data.
Having w’orked out the details of any required burn and checked them carefully. FIDO wrote all the information onto a standard form where about six copies would be made using carbonless copy paper. Capcom read the top copy up lo the spacecraft as pre-advisory data (PAD) – a list of numbers abbreviated down Lo barely the digits with units and decimal points omitted. Accuracy and interpretation was much easier when the PAD was laid out on a standard form. On board, the list was copied, usually by the LMP, onto an identical form and read back Lo Harth as a check of their accuracy. As the time for the burn approached, the data from the PAD was entered into the first of a series of programs on the computer that automatically controlled the burn. The PAD included three items that were crucial for the burn: when it should occur; the amount by which it should change the spacecraft’s velocity; and the direction in which the spacecraft should be pointing at the time of the burn. The PAD also included supplementary data: some was for the computer and its control routines; some was Lo improve the safely of the burn by offering details of backup methods of shutting down the engine; and some was to ensure that the attitude of the spacecraft was correct.
When flying in space, and especially when firing engines, it is of paramount importance to know in which direction a spacecraft is pointing. Three methods of cheeking this could be included in a typical PAD. A crewman could look through the sextant, having previously aimed it to a given angle, where he would expect a specific star to be centred in the eyepiece. A second check came from sighting another star with the COAS, having first mounted this sight in a window aimed in a known direction. However, their primary method of attitude determination relied on one of the major systems on board the spacecraft; the guidance and navigation (G&N) system with its inertial measurement unit (IMU) – a gyroscopically stabilised platform built into the spacecraft’s lower equipment bay below the optical systems. With the spacecraft aimed correctly and the PAD data entered into the computer, the burn could be controlled automatically or manually, as desired, with its results displayed for the crew Lo monitor.











 "I liked the toothpaste,” said Alan Bean.
"I liked the toothpaste,” said Alan Bean.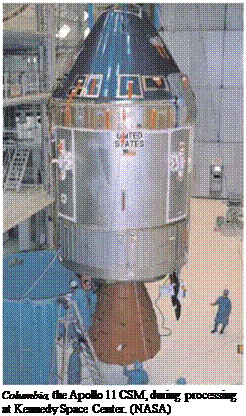 On the journey to the Moon, two events symbolised the crew’s daring and acceptance of risk more than any other. One was the landing itself, which committed two crewmen to stay forever on an utterly inhospitable lunar surface unless a small rocket engine worked properly to get them off and start their journey home to Earth. The other was lunar orbit insertion (LOI), the point in the journey when Apollo crews committed themselves to the gravity of the Moon. After LOI, there was no possibility of a return to Earth except by the successful operation of one major system within the service module. This was the service propulsion system (SPS), whose most obvious component was a large bell that protruded from the engine at the rear of the module. This engine, and the tanks that fed it, took up the bulk of the service module’s volume and mass, and its requirements largely defined the module’s layout and construction.
On the journey to the Moon, two events symbolised the crew’s daring and acceptance of risk more than any other. One was the landing itself, which committed two crewmen to stay forever on an utterly inhospitable lunar surface unless a small rocket engine worked properly to get them off and start their journey home to Earth. The other was lunar orbit insertion (LOI), the point in the journey when Apollo crews committed themselves to the gravity of the Moon. After LOI, there was no possibility of a return to Earth except by the successful operation of one major system within the service module. This was the service propulsion system (SPS), whose most obvious component was a large bell that protruded from the engine at the rear of the module. This engine, and the tanks that fed it, took up the bulk of the service module’s volume and mass, and its requirements largely defined the module’s layout and construction.